The next topic that we desire to discuss here is powers of i. Let's just take a look at what occurring while we start looking at many powers of i.
i1 = i i1 = i
i2 = -1 i2 = -1
i3 =i ⋅ i2 = -i i3 = -i
i4 = (i2 )2 = ( -1)2 = 1 i4 = 1
i5 = i ⋅ i4 = i i5 = i
i6 = i2 ⋅ i4 = ( -1) (1) = -1 i6 =-1
i7 = i ⋅ i6 = -i i7 = -i
i8 = (i4 )2 = (1)2 = 1 i8 = 1
Can you notice the pattern? All powers if i can be reduced down to one of four probable answers and they repeat every four powers continuously. It can be a convenient fact to remember.
Next we need to address an issue on dealing along with square roots of -ve numbers. we know that we can do the following.
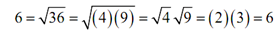
In other terms, we can break up products within a square root into a product of square roots provided both numbers are positive.
It turns out that actually we can do the same thing if one of the numbers is -ve. For instance,
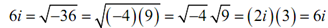
However, if both of numbers are negative it won't work anymore as the following shows.
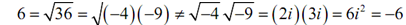
We can summarize it as a set of rules. If a & b are both positive numbers then,

Consider the following example to know it's important.