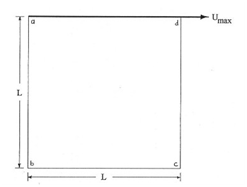
The figure shows a "square cavity flow". In this flow the cavity is filled with a fluid and the lid of the cavity moves with a constant velocity, while the other walls remain stationary. This causes a recirculation within the cavity. The flow is assumed 2 dimensional incompressible, with Umax=1.0 m/s constant. The lengths of the sides of the cavity, L, are all equal to 1.0 m

Run the program for the following Reynolds numbers: 10, 100, 500 and 1000.

The Reynolds number is defined by Re = Umax.L/n, where n is the fluid kinematic viscosity, which in this case, varies to produce the different

Reynolds numbers.

The boundary conditions are:

uab = ubc = ucd = vab= vbc= vcd = vad = 0 and uad = Umax

Use a staggered grid of at least 15x15 cells, and the SIMPLE algorithm for your solutions.

Plot both velocity and pressure distributions for all Reynolds numbers. Please submit your complete solution on either paper or electronically. If you choose paper, you must also send me the computer code electronically.