Munch Pressure Flow Model
Munch, a German plant physiologist, proposed in 1930, a simple physical model which can be tested in the laboratory for the mechanism of phloem transport. As shown in Figure, two osmometers, one containing solute at higher concentration .(A) than the other (B), are connected by a tube (C) and dipped in water. Due to high solute concentration in A water flows in to it by the process of osmosis. Consequently, the pressure develops which forces the solution to rise in the connecting tube and the content of A flow into 8. This pressure forces water to flow out of B through its membrane in the medium. In plants the state of source and sink is analogous to the two osmometers. The source regions have higher solute concentration than the sink region. The phloem conduit in the source region would imbibe water by the process of osmosis and generate a high turgor zone. On the other hand, the phloem in the sink region will be at low turgor. Thus, a pressure gradient exists along the length of the phloem which would cause a mass flow of solvent and solutes with equal velocity. There are several difficulties in accepting this, otherwise admirably simple and appealing hypothesis. Firstly, the generation of pressure gradient is possible in the above given model only if the water potential is about the same in both regions.
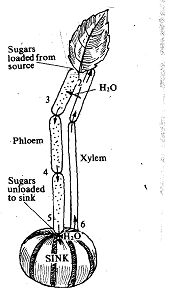
Figure: Munch Pressure Flow Model
However, in plants, water potential is lower in the Leaves and higher in the roots. Thus, the tendency of water to enter the phloem in the leaf region is reduced. Whereas in the root region, water can be readily imbibed by sieve elements even a lesser solute concentration of the phloem sap, from neighbouring cells and/or apoplasm. Therefore, it cannot be assumed that the gradient in solute concentration would generate a pressure gradient from sources to sinks. Moreover, the observed differential, mobilities of solute molecules, highest for K+ and lowest for ca2+, speak against the pressure flow hypothesis. The original model has been modified to remove this problem. P Pressure gradients sufficient for causing mass flow have not been recorded in any plant. Much depends, on the radius of the sieve plate pores, the presence of P-proteins and the degree of callose formation. Hence the debate on the Munch's models is still continuing, primarily because there is still no other alternative model to explain precisely the mechanism of Phloem transport. You may note that at the sites of loading and unloading there is active transport operating which may account. for the differential mobilities of ions and accumulation of molecules such as sugars in large amount against concentration gradient.