In financial analysis, interpolation is used widely in:
-
Determination of internal rate of return of a project.
-
Finding out the yield to maturity (ytm) of a bond or debenture.
-
Other situations where the time value of money is considered and interpolations have to be made while using the present and future value tables.
In financial analysis extrapolation is widely used for:
-
Forecasting future sales, cost and profit.
-
Long-term capital requirements.
-
Production of financial statements for financial institutions, banks, etc.Example 4
The cash inflows of a project involving an initial outlay of Rs.22 lakh is as follows:
|
Year
|
Rs. in lakh
|
1
2
3
4
|
10
10
6
3
|
The internal rate of return is the rate at which the total value of discounted cash outflows is exactly equal to the total value of discounted cash inflows. The internal rate of return of a project can be determined only through a process of trial and error.
To begin with, let us try the discount rate of 14%.
Using present value interest factor (PVIF) tables, the total of discounted cash inflows will be,
(10 x 0.877) + (10 x 0.769) + (6 x 0.675) + (3 x 0.592) = Rs.22.29 lakh.
Since this figure is higher than the initial outflow of Rs.22 lakh, we must discount at a higher rate.
At r = 15%, the total of discounted cash inflows will be,
(10 x 0.870) + (10 x 0.756) + (6 x 0.658) + (3 x 0.572) = Rs.21.93 lakh.
At the discount rate of 15%, the discounted cash inflows are slightly lower than Rs.22 lakh. It can be concluded that the internal rate of return must lie somewhere between 14% and 15%. The technique of interpolation can be used to determine the exact rate of return.
We now have a series of the following nature:
|
Rate%
|
Discounted Cash Flows (DCF)
(Rs. in lakh)
|
|
|
14
|
22.29
|
|
15
|
21.93
|
|
For an intermediary figure of Rs.22 lakh of discounted cash flow we need to interpolate the rate.
The linear approximation method may be used to interpolate. We know that when the rate increases by 1%, the DCF falls from 22.29 to 21.93 or the descent in DCF for 1% ascent in rate is (22.29 - 21.93). We also know that the interest rate must be higher than 14%, but less than 15%.
At the exact rate, the descent must be (22.29 - 22.00). When descent is
(22.29 - 21.93), the increase in rate is 1. For a descent of (22.29 - 22)
| the increase in rate must be |
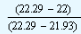 |
| The internal rate of return |
= |
14% + |
 |
| |
= |
14 + 0.806 ~ 14.81% |