Find the pure-strategy Nash equilibrium

Alice is on vacation in Wonderland and considers trying a special mushroom sold by the caterpillar. She cannot tell upfront if the mushroom is good or bad; she only discovers it later if it gives her a stomach ache. For the caterpillar, it costs more to sell good quality mushroom. The payoffs of Alice and the caterpillar are given in the table below:

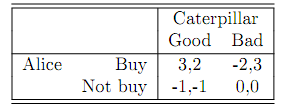
(a) A pure strategy is a strategy that does not involve randomization. Find the pure-strategy Nash equilibrium/equilibria of this game.

(b) Alice likes Wonderland so she decides to stay there for 10 days: the above game is repeated 10 times. Can you find a trigger strategy that induces Buy/Good for all 10 periods? How about the first 9 days?

(c) Alice falls in love with Wonderland and decides to live there for the rest of her life. The above game is thus repeated infinitely. If the interest rate is zero, explain what trigger strategy will induce the cooperative Buy/Good equilibrium.

(d) Assume now that the interest rate is greater than zero, and the game is repeated infinitely. Find a range of r such that the cooperative equilibrium can be sustained.

(e) It is possible that Alice will have to leave Wonderland and return home eventually. More specifically, each period players think that there is a 30% chance that the game does not continue next period. Is the cooperative equilibrium still possible to maintain? If yes, for what values of the interest rate? If not, why not?