Q. Example of inference?

Ans.

Thirty students from a college were surveyed and classified according to two variables: (1) gender (M/F) and (2) major (liberal arts, business administration, technology). The results of the survey are shown in this chart and histogram.

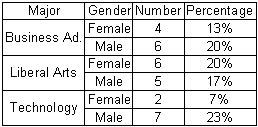
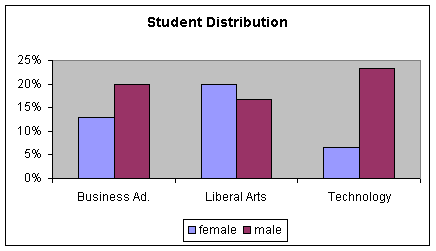
What inferences might you make based on this data?
What precautions should you have about making these inferences?

Solution:

1. Inference:

You might make the following inferences from the sample data:

A. The most drastic difference in the above histogram seems to be in the percentage of females majoring in technology compared to the percentage of males in technology. About 23% of the people sampled were male and in technology whereas only 7% of the sample population was female and in technology. From this data, you might deduce that there are over 3 times as many male technology majors as there are female technology majors in the college.

B. Another inference you might make, based on the comparison of gender is that liberal arts has a smaller gender divide than both other major areas because there seem to be an almost equal number of males and females who are majoring in liberal arts.

C. Look at the total number of people in the sample majoring in the different subject areas. 33% of the sample are majoring in business administration, 37% are in liberal arts and 30% are in technology. You might infer that these percentages reflect the entire college student population too.

D. You might use this information to predict the choice of majors for freshmen based on their genders. It seems much more likely that a female freshman would choose to major in business administration or liberal arts than in technology.

E. Notice that the sample population was 40% female and 60% male. You might infer from this that the college has 60% male and 40% female students, too.

2. Precautions:

A. Is this sample a good representation of the school? How was the sample chosen? Were all the students in the library at one time, in the lobby of a building, in the student center? What time was the survey done? Was it early in the morning or late in the evening? Was there any effort to get a representative mix of years? Were all students in the sample in their senior year?

B. Is this sample (30 students) large enough to conclude anything accurately about the entire college population? The larger a sample population, the more accurately the sample data reflects the population data. This is often a serious concern among statisticians. How big is big enough.