In the shape of a cone a tank of water is leaking water at a constant rate of 2 ft3 /hour . The base radius of the tank is equal to 5 ft and the height of the tank is 14 ft.
(a) At what rate is the depth of water of the tank changing while the depth of the water is 6 ft?
(b) At what rate is the radius of the top of water of the tank changing while the depth of the water is 6 ft?
Solution : We have to probably start off with a quick sketch (probably not to scale) of what is going on.
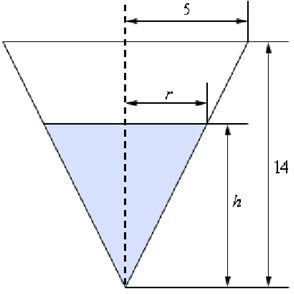
As we can illustrate, the water of the tank in fact forms a smaller cone along with the similar central angle like the tank itself. At any time the radius of the "water" cone is specified by r and height of the "water" cone at any time is specified by h. The volume of water of the tank at any time t is specified by,
V = 1/3 ∏ r 2 h
and we've been specified that V ′ = -2 .
(a) At what rate is the depth of the water of tank changing while the depth of the water is 6 ft?
For this part we have to determine h′ while h = 6 and now we have a problem. The only formula which we've got which will associate the volume to the height also involves the radius and thus if we were to differentiate this w.r.t. t we would get,
V ′ = 2/3 ? rr′h + 1/3∏ r 2 h′
Thus, in this equation we know V ′ & h and desire to find h′ , however we don't know r & r′ . As we'll illustrates finding r isn't too bad, however we just don't have sufficient information, at this point which will let us to find r′ and h′ simultaneously.
To fix this we'll have to remove the r from the volume formula in some way. This is in fact easier than it may at first look. If we go back to our sketch above & look at just the right half of the tank we illustrates which we have two similar triangles and while we say same we mean similar in the geometric sense. Recall that two triangles are called alike if their angles are similar, which is the case here. While we have two same triangles then ratios of any two sides will be equivalent. For our set it means that we have,
r /h= 5/14 ⇒ r = 5/14 h
If we take it and plug it into our volume formula we have following,
V= 1/3 ∏ r 2 h = 1 /3 ∏ ( 5 /14) h) )2 h = 25/588 ∏ h3
It gives us a volume formula which only involved the volume & the height of the water. However, note as well that this volume formula is just valid for our cone, thus don't be tempted to utilize it for other cones! Now if we differentiate this we have,
V ′ = 25/196 ∏ r 2 h'
At this point all we required to do is plug in what we know and solve out for h′ .
-2 = (25/196) ∏ (62 ) h′ ⇒ h′ = (-98 /225 ∏) = -0.1386
Thus, it looks like the height is reducing at a rate of 0.1386 ft/hr.
(b) At what rate is the radius of the top of the water of tank changing while the depth of the water is 6 ft?
In this instance we are asking for r′ and there is simple way to do this part and a hard (well, more hard than the easy way anyway....) way to do it. The "difficult" way is to redo the work in part (a) above just this time use,
h /r= 14/15 ⇒ h = (14/5) r
to acquire the volume in terms of V & r and then proceed as before.
That's not terribly hard, however it is more work that we have to so. Recall from the first part which we have,
r = 5/14 h ⇒ r′ =5/14 h'
Thus, as we can illustrated if we take the relationship which relates r & h that we utilized in the first part & differentiate it we get a relationship among r′ & h′ . At this instance all we have to do here is utilizes the result from the first part to get,
r′ = ( 5 /14)(-98/225 ?)= - 7/45 ∏ = -0.04951
Much simple that redoing all of first part. Note though, that we were just able to do it the "easier" way since it was asking for r′ at precisely the similar time that we asked for h′ in the first part. If we hadn't been by using the similar time then we would have had no option but to do this the "difficult" way.
In order to determine the asked for rate all we required is an equation which relates the rate we're looking for to rate that we know already. Occasionally there are multiple equations which we can utilizes and sometimes one will be simpler than another.
Also, this problem illustrated us that we will frequently have an equation which contains more variables which we have information regarding and so, in these cases, we will have to remove one (or more) of the variables. In this problem we removed the extra variable by using the idea of same triangles. It will not always be how we do this, but several of these problems do utilizes similar triangles so ensure you can utilizes that idea.