Now that we have an understanding about price volatility characteristics of a bond, let us turn to the duration/convexity approach, which is an alternative approach to full valuation. Duration is the change in the value of bond that will result from a hundred basis point change in yield. Since this approach uses both

measures - duration and convexity, it is named as duration/convexity approach. Duration is the first approximation of the percentage change in price. Convexity is used to improve the estimate provided by duration.

Duration of the bond is calculated as follows:

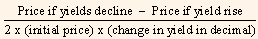
If we assume,

         Δy = Change in yield in decimal.

         V₀ = Initial price.

         V_- = Price if yields decline by Δy.

         V₊ = Price if yields increase by Δy.

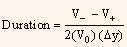                                                                                            ...Eq.(1)

For example, consider an 8.5% coupon 20-years option-free bond selling at Rs.115.89 to yield 7% (refer table 9). Let's assume that there are both side changes in the yield by 25 basis points. After the increase of 25 basis points from 7.00% to 7.25%, the price decreases to Rs.112.99.  When yield decreases by 25 basis points from 7.00% to 6.75%, the price increases to Rs.118.91.

Substitute values in Eq. (1) to get the duration of the bond as given below:

         Δy = 0.0025

         V₀ = Rs.115.89

         V_- = Rs.118.91

         V₊ = Rs.112.99

Then,

         Duration =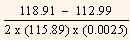 

         Duration = 10.22

Duration of 10.22 means that the there will be 10.22% change in the price of this bond for a 100 basis point change in yield.