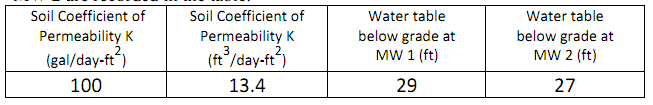
A construction project involves excavation below grade. A 24 inch diameter dewatering well is installed from grade (EL 586) to 80 ft below grade to lower the water table. Soil borings of the site have determined that bedrock is located at EL 440. Two monitoring wells (MW 1 and MW 2) have determined the water table in the construction zone to be at EL 574 before pumping. MW 1 is located 150 ft from the well and MW 2 is located 285 ft from the well. After steady pumping, the water table levels below grade at MW 1 and MW 2 are recorded in the table.
Based on the data provided, the flow rate from the dewatering well is closest to:
Solution: This is an unconfined aquifer problem. Use the Dupuit equation.
Q = pK((h2)2 - (h1)2)/ln(r2/r1) Q = ??((h2)2 - (h1)2)/((1055)log(r2/r1))
Step 1: Use K in ft3/day- ft2 (because the units of the answer are in ft3/day)
Step 2: Determine the vertical and horizontal linear distances.
Aquifer thickness = EL 574 - EL 440 = 134 ft
Determine h1: EL 586 - 29 = EL 557; EL 574 - EL 557 = 17 ft
h1 = aquifer thickness minus the drawdown = 134 - 17 = 117 ft
r1 =150 ft
Determine h2: EL 586 - 27 = EL 559; EL 574 - EL 559 = 15 ft
h2 = aquifer thickness minus the drawdown = 134 - 15 = 119 ft
r2 = 285 ft
Step 3: Solve for Q Q = pK((h2)2 - (h1)2)/ln(r2/r1) Q = 13.4p((119)2 - (117)2)/ln(285/150)
Answer is: Q = (42(14,161 - 13,689))/0.64 = 30,975 ft3/day