Describe the Types of triangles ?

Triangles can be classified according to the lengths of the sides or the measures of the angles.

1. Naming triangles by sides

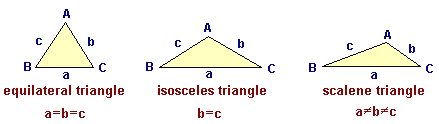
An equilateral triangle is a triangle with three congruent sides.
An isosceles triangle is a triangle with at least two congruent sides.
A scalene triangle is a triangle with no congruent sides.

In an isosceles triangle DABC, if @ , and are called legs of the isosceles triangle,  B and  C are called base angles and  A is called the vertex angle.

2 Naming triangles by angles

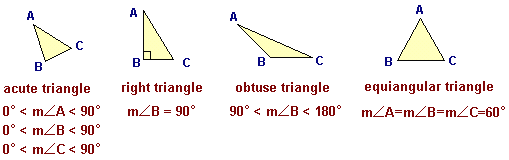
An acute triangle is a triangle with three acute angles.
A right triangle is a triangle with a right angle.
An obtuse triangle is a triangle with an obtuse angle.
An equiangular triangle is a triangle with three congruent angles.

In a right triangle, the side opposite the right angle is called the hypotenuse, the sides that form the right angle are called legs.

Exercise 5.1

Name the legs, base angles and vertex angle in DDEF. Assume that
Name the legs and hypotenuse in DGHK. Assume that mH = 90.

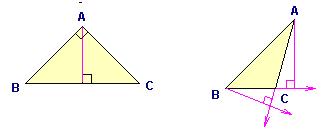
Definition 5.1

An altitude of a triangle is a segment from a vertex to a point on the opposite side (perhaps extended) and the segment is perpendicular to the opposite side.

Note that in a right triangle two of the altitudes coincide with the legs of the right triangle. In an obtuse triangle two altitudes have feet on the extension of the sides.

Definition 5.2

A median of a triangle is a segment drawn from a vertex of a triangle to the midpoint of the opposite side.