Describe Segments, Rays, Angles, and Triangles
We now define some more basic geometric figures.
1. Segments
Definition
A segment is the set of two given points and all the collinear points between them.

2. Rays
Definition
A ray is a part of a line. It begins at a given point (endpoint) and then extends infinitely in only one direction. It is the set containing the endpoint and all points on one side of the endpoint.
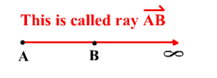
We denote A and B as . The first letter is the endpoint of the ray, the second is any point other than the endpoint on the ray.
Definition
Opposite rays are rays that have a common endpoint and which form a line.
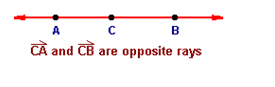
3. Angles
Definition
An angle is made up of two rays with a common endpoint. This endpoint is called the vertex of the angle. The sides of the angle are the rays.
We use the symbol "?" to denote an angle.
We can name an angle in several ways. The above angle can be named as ?1, ?B, ?ABC or ?CBA. In naming angles with a single letter, the letter of the vertex must be used. Notice that the letter of the vertex should be in the middle if we name an angle by three letters.
4. Triangles
Definition
A triangle is the union of three segments determined by the three noncollinear points. The segments are the sides of the triangle. A vertex of a triangle is the intersection of any two sides.

We use the symbol "D" to denote a triangle. A triangle with vertices A, B, and C can be named as DABC, DACB, DBAC, DBCA, DCAB or DCBA.