Calculation of tax benefits of capital allowances

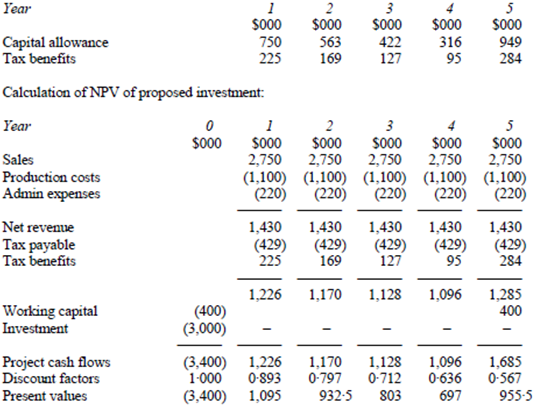
The net present value is approximately $1083000

An alternative solution using annuity factors is as follows.

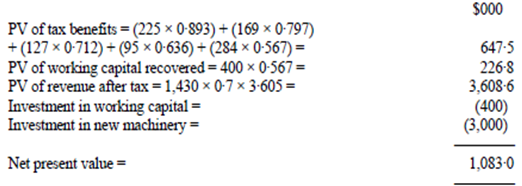
The net present value is approximately $1083000

This analysis formulates the following assumptions

(1) The first tax benefit takes place in Year 1 the last tax benefit occurs in Year 5

(2) Cash flows take place at the end of each year.

(3) Inflation is able to be ignored.

(4) The increase in capacity doesn't lead to any increase in fixed production overheads.

(5) Working capital is all out at the end of Year 5

(b)

Distribution and Administration expenses per unit = 220000/5500 = $40 per unit

Net revenue as of additional units sold = 500 - 200 - 40 = $260 per unit

Present value of tax benefits = $647500

Incremental working capital per unit = 400000/5500 = $72·73 per unit

Let annual sales volume be SV units

NPV = [SV × 260 × (1 - 0·3) × 3·605] + 647,500 - [72·73 × SV × (1 - 0·567)] - 3,000,000 = 0

Thus SV = (3,000,000 - 647,500)/ (656·11 - 31·49)  = 2,352,500 /624·62 = 3,766 units Annual increase in sales volume of 3,766 units will produce a zero NPV

This is 31% (100 × 1,734/5,500) below the expected increase in sales volume. (Note: working capital is supposed to depend on sales volume)