Calculate the greatest stress - plate:

A rectangular plate 10 mm thick within a hole of 50 mm diameter drilled on this is as shown in Figure 10. This is subjected to axial pull of 45 kN. Calculate the greatest and the least intensities of stress at the critical cross-section of the plate.

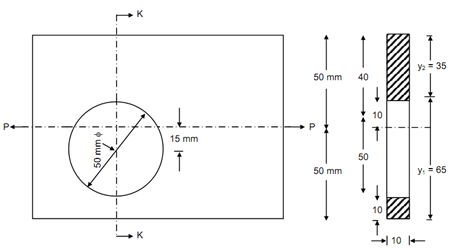
Critical Section along K - K

Figure

Solution

Area of section at the weakest point, A = (10 × 10) + (40 × 10) = 500 mm2 .

To situate the centroidal axes, taking moments around AB,

y₁  = ((10 × 10) + (40 × 10 × 80)) /((10 × 10) + 40 × 10))= 65 mm bottom

Therefore,   y₂  = 100 - 65 = 35 mm

Moment of inertia around xx-axis,

I _xx       = (10 × 40³ /12) + 40 × 10 × (35 - 20)²  + (10 × 10³ )/ 12+ 10 × 10 × (65 - 5)²

= 50.42 × 10⁴  mm⁴

Axial load, P = 45 kN = 45 × 103 N  (tensile)

Direct stress, f₀  = P    =45 × 10³/500 = 90 N/mm² (tensile)

Eccentricity, e = 65 - 50 = 15 mm

Bending stress along edge, AB = (P × e/ I _xx) × y

=(45 × 10³ × 15) / (50.42 × 10⁴) × (- 65)

=- 87 N/mm² (compressive)

Bending stress along edge, CD = ( 45 × 10³ × 15)/ (50.42 × 10⁴)  × (35)

                                                 = 46.8 N/mm² (compressive)

Maximum stress along edge, AB =- (90 + 87) = - 177 N/mm2 (tensile)

Minimum stress along edge, CD =- 90 + 46.8 = - 43.2 N/mm2(tensile).