Question:
Unsatisfactory control of spare parts in a particular mechanical workshop is resulting in high carrying costs for some items and high stock-out costs for others. A study of the past demand and order lead time patterns for a particular item, X, gives the following information:

Probability of a lead time of 2 days is 0.10
Probability of a lead time of 3 days is 0.26
Probability of a lead time of 4 days is 0.40
Probability of a lead time of 5 days is 0.24
A lead time of x days means the order is placed at the end of the day that stock runs out and is received after x full days.
A proposal has been made that a policy of ordering a basic 35 units should be adopted whenever stock falls below 30 units. To the order of 35 units there should be added the number of units necessary to bring stock up to the re-order point of 30 units at the time the order is made.
A stock of 40 units is in hand. Ordering costs amount to $25 per order, carrying costs are $1 per day and stock-out costs are $4 per unit. Stock-out costs are incurred automatically whenever there is no stock in store, since the stock has to be procured immediately from an outside source.
The workshop administrators decided to carry out a Monte Carlo simulation of the demand for the item X.
Random numbers for 10 days of the demand sequence are given below:
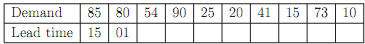
(a) Carry out the simulation of the 10 days' demand on stock levels and calculate the average daily demand from the simulation.
(b) Compare the original expected daily demand with the average daily demand obtained from the simulation.
(c) Calculate the average daily stock cost.