Calculate the angular acceleration of the pulleys:
The compound pulley system has a mass of 30 kg and a radius of gyration of 450 mm. Calculate the tension in each cord and the angular acceleration of the pulleys when the masses are released (Refer following Figure).
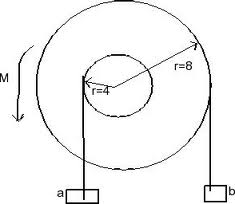
Solution
Free body diagram for the pulley and both the masses are drawn separately as shown in the figure.
We must keep in mind that a1 = 0.6 α and a2 = 0.3 α acceleration (i.e. difference in radii). where α is the angular
Inertial forces are illustrated on FBD. Now, we may write down dynamic equilibrium equations for each of the body.
For a mass of 50 kg, we have
∑ Fy = 0 ∴ T1 - 50 a1 - 50 × 9.81 = 0
Substituting for a1 in terms of α, we get
T1 = 50 × 0.6 α + 50 × 9.81
= 490.5 + 30 α ------------- (1)
For the mass of 150 kg, we have
∑ Fy = 0 ∴ T2 + 150 . a2 - 150 × 9.81 = 0
∴ T2 = 1471.5 - 150 × 0.3 α
= 1471.5 - 45 α ---------- (2)
For the pulley, we have ∑ M = 0
∴ 0.6 T1 + I α - 0.3 T2 = 0
But I = 30 × (0.45)2 = 6.08 kg m 2
∴ 0.6 T1 - 0.3 T2 = - 6.08 α ---------- (3)
Solving Eqs. (1), (2) and (3) as below, we obtain
0.6 T1 - 0.3 T2 = - 6.08 α
0.6 (490.5 + 30 α) - 0.3 (1471.5 - 45 α) = - 6.08 α
or, 147.15 = 37.58 α
α = 3.91 rad / sec.
Substituting this value of α in Eq. (1) and (2) above we obtain
T1 = 607.8 N
and T2 = 1295.55 N.