Reference no: EM13271268

Question 1

Howard Bowen is a large-scale cotton farmer. The land and machinery he owns has a current market value of $4 million. Bowen owes his local bank $3 million. Last year, Bowen sold $5 million worth of cotton. His variable operating costs were $4.5 million; accounting depreciation was $40,000, although the actual decline in value of Bowen's machinery was $60,000 last year. Bowen paid himself a salary of $50,000, which is not considered part of his variable operating costs. Interest on his bank loan was $400,000.

If Bowen worked for another farmer or a local manufacturer, his annual income would be about $30,000. Bowen can invest any funds that would be derived, if the farm sold, to earn 10 percent annually. (Ignore taxes.)

a. Compute and interpret Bowen's accounting profits.

b. Compute and interpret Bowen's economic profits. 

Question 2

The Blair Company's three assembly plants are located in California, Georgia and New Jersey. Previously, the company purchased a major subassembly, which becomes part of the final product, from an outside firm. Blair has decided to manufacture the subassemblies within the company and must now consider whether to rent one centrally located facility (e.g., in Missouri, where all subassemblies would be manufactured) or to rent three separate facilities, each located near one of the assembly plants, where each facility would manufacture only the subassemblies needed for the nearby assembly plant. A single, centrally located facility, with a production capacity of 18,000 units per year, would have fixed costs of $900,000 per year and a variable cost of $250 per unit.

Three separate decentralised facilities, with production capacities of 8,000, 6,000 and 4,000 units per year, would have fixed costs of $475,000, $425,000 and $400,000, respectively, and variable costs per unit of only $225 per unit, owing primarily to the reduction in shipping costs. The current production rates at the three assembly plants are 6,000, 4,500 and 3,000 units, respectively.

a. Assuming that the current production rates are maintained at the three assembly plants, which alternative should management select? Justify your answers.

b. If demand for the final product were to increase to production capacity, which alternative would be more attractive? Why?

c. What additional information would be useful before making a decision? Discuss.

Question 3

Consider the following payoff matrix:

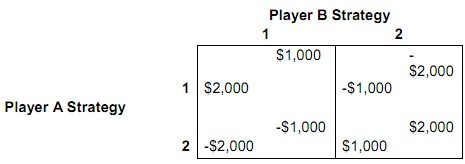
a. Does Player A have a dominant strategy? Explain why or why not.

b. Does Player B have a dominant strategy? Explain why or why not.

Question 4

a. Ethanol is again viewed as one part of a solution to the problem of shortages of petroleum products. Ethanol is made from a blend of gasoline and alcohol derived from corn or sugar cane. What would you expect the impact of this program to be on the price of corn, soybeans and wheat? Discuss.

b. Why invest capita in purely competitive industries with equilibrium margins that are razor thin and entrants that erode quasi profits? Suppose volume is not exceptionally large, why then?

Question 5

The amount of fish caught per week on a trawler is a function of the crew size assigned to operate the boat. Based on past data, the following production schedule was developed:

a. Over what ranges of workers are there (i) increasing, (ii) constant, (iii) deceasing and (iv) negative returns?

b. How large a crew should be used if the trawler owner is interested in maximising the total amount of fish caught?

c. How large a crew should be used if the trawler owner is interested in maximising the average amount of fish caught per person?

d. Supposed the owner of the trawler can sell all the fish caught for $75 per 100 pound and can hire as many crew members as desired by paying them $150 per week. Assuming that the owner of the trawler is interested in maximising profits, determine the optimal crew size.

Question 6

Assume that a firm in a perfectly competitive industry has the following total cost schedule:

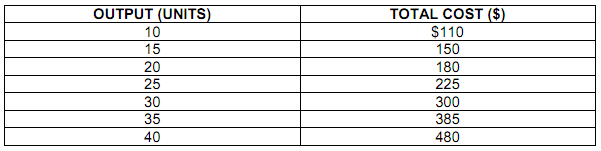
a. Calculate a marginal cost and an average cost schedule for the firm.

b. If the prevailing market price is $17 per unit, how many units will be produced and sold? What are profits per unit? What are total profits?

c. Is the industry in long-run equilibrium at this price? Explain.

Question 7

Consider the following short-run production function (where L = variable input, Q = output):

Q = 6L³- 0.4L³

a. Determine the marginal product function (MPL).

b. Determine the average product function (APL).

c. Find the value of L that maximises Q.

d. Find the value of L at which the marginal product function takes on its maximum value.

e. Find the value of L at which the average product function takes on its maximum value. 

Question 8

The Lumins Lamp Company, a producer of old-style oil lamps, estimated the following demand function for its product:

Q = 120,000 - 10,000P

where Q is the quantity demanded per year and P is the price per lamp. The firm's fixed costs are $12,000 and variable costs are $1.50 per lamp.

a. Write an equation for the total revenue (TR) function in terms of Q.

b. Specify the marginal revenue function.

c. Write an equation for the total cost (TC) function in terms of Q.

d. Specify the marginal cost function.

e. Write an equation for total profits (π) in terms of Q. At what level of output (Q) are total profits maximised? What price will be charged? What are total profits at this output level?

f. Check your answer in Part (e) by equating the marginal revenue and marginal cost functions, determined in Parts (b) and (d), and solving for Q.

g. What model of market pricing behavior has been assumed in this problem? Explain.