Reference no: EM131314700

Trigonometry and Calculus

Questions 1-20: Select the one best answer to each question.

1. As a lumberjack, you attach a 50-foot wire from the top of a lean¬ing pine tree to a stake in the ground, forming a 70 degree angle with the ground. You then measure the distance along the ground from the stake to the center of the tree's base. To determine the length of the tree, what law or theorem should you use?
A. Law of Sines
B. Law of Cosines
C. Pythagorean Theorem
D. Quadratic Formula

2. Suppose a table is 3 feet long and 7 feet wide. The distance between opposite corners is
A. 2.6 feet.
B. 3.7 feet.
C. 7.6 feet.
D. 10.0 feet.

3. The derivative of y = x²- 3x at the point (0, 0) is
A. -3.
B. 0.
C. 1.
D. 3.

4. Suppose you have 240 feet of fencing with which to build three connected equally sized pens as shown below, so as to maximize the area bounded.

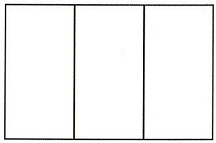
What will be the total area of the three pens?
A. 1200 square feet
B. 1800 square feet
C. 2000 square feet
D. 2400 square feet

5. In problem 4 above, what will be the dimensions of each pen?
A. 60 by 30 feet
B. 20 by 60 feet
C. 20 by 30 feet
D. 20 by 40 feet

Use the following information to answer Questions 6 and 7.

Suppose a right triangle ABC with its right angle at C has a 25 degree angle at A and the opposite side a has length 7.

6. The angle at B has a measure of
A. 55 degrees.
B. 60 degrees.
C. 65 degrees.
D. 70 degrees.

7. What is the length of the hypotenuse rounded to one decimal place?
A. 16.6
B. 16.5
C. 7.8
D. 7.7

8. Determine the x coordinate of the point where the derivative of y = x² - 2x equals 0.
A. - 2
B. -1
C. 1
D. 2

9. While fishing on a local lake, you see a fish below water at a 25 degree angle with the surface, producing air bubbles on the surface of the water 20 feet ahead. How far away is the fish from the boat (rounded to one decimal place)?
A. 9.3 feet
B. 18.1 feet
C. 22.1 feet
D. 47.3 feet

10. If k(x) = x² - 3x, the value of k(3 + h) is
A. h² + 9h.
B. 4h.
C. 3h + h².
D. h² + h.

11. A road sign at a lookout reads: "5% grade next 4 miles." What is the change in elevation from the lookout to the point four miles ahead (to the nearest foot)? Recall that there are 5280 feet in a mile.
A. 21,146 feet
B. 21,094 feet
C. 1069 feet
D. 1055 feet

12. You estimate the area of a large spot on your carpet by covering the spot with rectangular strips of tissue paper. If your strips are 4 inches wide and a total of 12 feet long, what is a reasonable estimate of the spot's area?
A. 48 square feet
B. 12 square feet
C. 4 square feet
D. 2 square feet

13. Suppose a triangle ABC with an 80 degree angle at A has a 40 degree angle at B and the side b has length 4. Determine the length of the side a (rounded to one decimal place).
A. 6.1
B. 3.9
C. 2.6
D. 2.5

14. Find the derivative of the function f (x) = x² + 5x.
A. 
B. 2x
C. 5x + 2
D. 2x + 5

15. You're in a hot-air balloon with your support crew on the ground. Attached to the balloon are two tether cords of length 350 feet and 400 feet that your crew attached to the ground. You note that these cords form an angle of 50 degrees where they meet the balloon. Assuming these lines are taut and the ground is level, what is the distance between the points on the ground where the two tether cords are attached (rounded to one decimal place)?
A. 531.5 feet
B. 338.1 feet
C. 320.2 feet
D. 220.1 feet

16. If f (x) = 2x - 5, the value of f(4) is
A. 3.
B. 5.
C. 8.
D. 13.

17. From a point 50 meters from the bottom of a radio tower along level ground, the angle of elevation to the top of the tower is 67 degrees. The height of the tower is
A. 54.3 meters.
B. 117.8 meters.
C. 127.9 meters.
D. 128 meters.

18. An example of a function whose derivative is always 3 is
A. f (x) = x + 3.
B. f (x) = 5x + 3.
C. f (x) = 3x+ 5.
D. f (x) = 3.

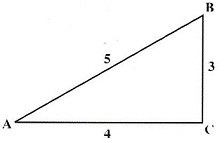
19. In the above sketch, sin B has a value of
A. 4/3.
B. 415.
C. 3/4.
D. 3/5.

20. In the above sketch, tan B has a value of
A. 3/5.
B. 3/4.
C. 4/5.
D. 4/3.