What is the coefficient of restitution for the collision

Reference no: EM13796218

1. A wooden plank is placed horizontally on the floor. A wedge of wood (object A, m_A = 10kg), cut with an angle of Φ = 20°, is placed on top of the plank. A block of wood (object B, m_B = 20kg) is placed on top of the wedge. Both objects and the plank are initially at rest, and the coefficients of static friction between the surfaces are µ_s,AB = 0.7 and µ_s,pA = 0.3, respectively. One end of the plank is very slowly raised up off the floor (θ starts at 0° and increases slowly from there).

a. Assuming that object B doesn't slide on object A, at what angle (θ) will the combined object AB begin to slide?

b. Assuming that object A doesn't slide on the plank while object B is free to slide, at what angle (θ) will object B begin to slide?

c. From the formulas used to calculate parts (a) and (b), derive a formula defining the necessary relationship between the values of µ_s,AB and µ_s,PA such that both A and B would begin to slide at the same angle (θ)?

2. Block 1 is placed on a 30° incline, and is attached to block 2 by a rope. Block 2 is hanging from a pulley over the top edge of the incline and is also completely submerged in a tank of water.

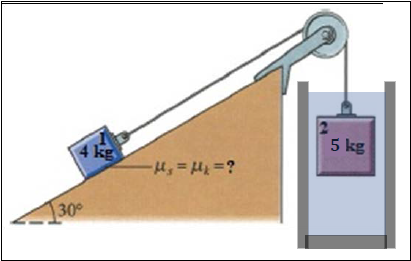
a. For what block 2 density could static equilibrium be maintained without static friction?

b. If block 2 were aluminum, what is the smallest coefficient of static friction required to for static equilibrium?

c. If the coefficient of static friction were µ_s = 0.30, for what maximum and minimum block 2 densities could static equilibrium be maintained?

3. Given the vector relationship and vector definitions below, find the magnitude and direction of E^→.

A^→- B^→+½C^→+1/3(E^→ - D^→) = 0

A^→= 2x^ + 5y^ B^→ = (7, -4) C^→= 9 at 210° D^→= 12 at 25° above - x^

4. A 480 kg car moving at 16.2 m/s hits, from behind, another car of mass 570 kg moving at 13.3 m/s in the same direction. The second car's new velocity is 15.1 m/s.

a. What is the coefficient of restitution for the collision?

b. What percentage of the kinetic energy is lost in the collision?

c. With what speeds would the cars rebound if the collision was perfectly elastic?

Reference no: EM13796218

Questions Cloud

Demands resulting from the influx in population : What were the major forces behind urban growth in early United States history? What influence did urban growth have on urban concentration and population density? What new demands were placed on city governments when population density increased..

Analysis the number of buyers and their relative sized : Analysis the number of buyers and their relative sized.

Political system reflect the distribution of power : How is power distributed in the U.S. political system? How does the U.S. political system reflect the distribution of power? List and discuss six ways in which urban planning can be political in nature.

Describe the five phases of a project : Determine which project might be implemented and why (e.g. feasibility study, breakeven analysis, etc). Describe the five phases of a project

What is the coefficient of restitution for the collision : A 480 kg car moving at 16.2 m/s hits, from behind, another car of mass 570 kg moving at 13.3 m/s in the same direction. The second car's new velocity is 15.1 m/s. What is the coefficient of restitution for the collision

Define challenges in the global business environment : Challenges in the global business environment are mostly attributed to unethical business practices, failure to embrace technology advancements, and stiff competition among businesses

Analyzing cultural relevance in instruction : Analyzing Cultural Relevance in Instruction

Problems based on complex exponentials : Difference Equations: Solve the following difference equation using recursion by hand (for n=0 to n=4)

Environmental planning-political theories : List and discuss the reasons why environmental planning can be difficult to pursue? How does federal environmental planning influence state and local environmental planning?

User Account

All Pages