Reference no: EM13720053

Questing 1: Pine has density ρ_p = 0.373 x 10³ kg/m³. The density of seawater is ρ_w = 1.03 x 10³ kg/m³. What fraction of a pine log floating in seawater is below the surface?

Question 2: A cube a³ is suspended in liquid of density ρ. The top and bottom faces of the cube are horizontal. Use the difference in pressure between the top and bottom faces to calculate the buoyant force on the cube, and show that this buoyant force is equal to the weight of the water displaced by the cube.

Question 3: A steel ball has radius r = 12.5 mm and mass m = 67 g. What would be the apparent weight of this ball under water?

Question 4: A rubber chicken is hung from a spring, which stretches a distance x as a result. Then the rubber chicken (still suspended from the spring) is submerged in water, and the spring stretch is measured to be only 10% of the initial stretch x. What is the density of the rubber chicken? Hint: m_rc = ρ_rcν.

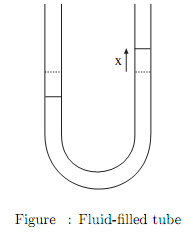
Question 5: A u-shaped tube, with uniform internal radius r, is partially filled with a liquid of density ρ. (Figure) The total length of liquid-filled tube is L. If the liquid level is somehow disturbed from its equilibrium position, it will oscillate. Show that the oscillation is simple harmonic, and find the angular frequency ω of the oscillation. (Assume that any viscous damping forces are negligible.)

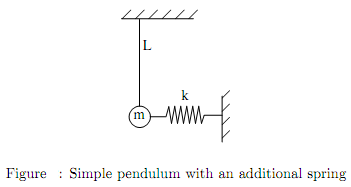
Question 6: A simple pendulum of length L and mass m is attached to a horizontal spring with spring constant k as shown in figure. Show that for small oscillations, the motion of the mass is approximately simple harmonic, and determine the angular frequency ω of the oscillation.

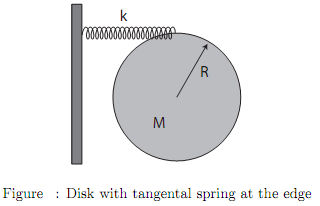
Question 7: A uniform disk is mounted on a low-friction bearing at its center. A spring is attached to the edge of the disk, so that the spring is in equilibrium when it is tangental to the edge of the disk, as shown in figure. Show that for small oscillations of the disk ("small" meaning that sin θ = θ and cos θ = 1) the motion of the disk is simple harmonic, and find the angular frequency ω. The rotational inertia of a disk about the center is i = ½ MR².

Question 8: A simple pendulum is a point mass on a mass less string, and T_s = 2Π √L/g. A physical pendulum formed by a small mass on a lightweight string is approximately a simple pendulum, but not quite; its period is T_p = 2Π√1/mgL. Is the period of a physical pendulum longer or shorter than the period of a simple pendulum with the same length? Assume L is measured to the center of mass, and justify your answer.

Question 9: You wish to build your own pendulum clock. The first thing you will need is a pendulum with a period of 2.00 seconds. Assuming that a simple pendulum is an appropriate approximation, what should be the length of your pendulum?

Question 10: You're still trying to build a pendulum clock. You don't have a simple pendulum available, since point masses are actually quite difficult to attach to mass less strings, so you're using a uniform rod instead. You still need the period to be 2.00 seconds. What should be the mass and length of the bar, which pivots at one end?

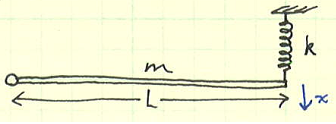
Question 11: A horizontal stick has a pivot on the left end and is supported by a spring on the right end. Find the angular frequency ω for the stick, for small oscillations.