What do the constitutive equations tell you about

Reference no: EM132172120

Problem 6.1

The problem show within the box was worked incorrectly by an MIT student on an exam. The student's work is shown immediately below the problem statement, again with the box.

i) Find and describe the error.

ii) Re-formulate the problem-that is, construct a set of equations from which you might obtain valid estimates for the forces in the two supporting members, BD and CD.

A rigid beam is supported at the three pins, A,B, and C by the wall and the two elastic members of common material and identical cross-section. The rigid beam is weightles but carries an end load W Find the forces in the members BD and CD in terms of W.

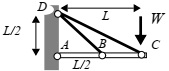
Problem 6.2

The rigid beam is pinned at the left end and supported also by two linear springs as shown.

What do the equilibrium requirements tell you about the forces in the spring and their relation to P and how they depend upon dimensions shown?

Assuming small deflections (let the beam rotate cw a small angle θ), what does compatibility of deformation tell you about the relationships among the contractions of the spring, the angle θ?

What do the constitutive equations tell you about the relations between the forces in the springs and their respective deflections?

Express the spring forces as a function of P if k₂ = (1/4)k₁

Problem 6.3

All members of the truss structure shown at the left are of the same material (Elastic modulus E), and have the same cross sectional area. Fill in the elements of the stiffness matrix.

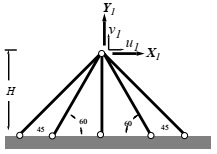
Problem 6.4

The truss show below is loaded at midspan with a weight P= 60 lbs. The member lengths and cross sectional areas are given in the ?gure. The members are all made of steel.

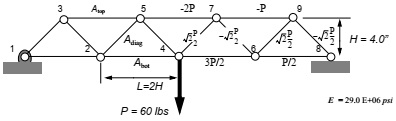
a) Verify that the forces in the members are as indicated.

b) Using Trussworks, determine the vertical deflections at nodes 2 and 4.

Reference no: EM132172120

Questions Cloud

Control process and provide example for each : Describe the four steps that the company would use in the control process and provide an example for each.

Today insurance market affect hospitals : What happens to hospitals if the cost of healthcare becomes unaffordable and how does today’s insurance market affect hospitals?

Have you ever wondered why you behaved in a certain way : Have you ever wondered why you behaved in a certain way? Or why you felt a certain way about a situation? The unique ways you think, act and feel throughout.

Shell case fabricators designs and builds shell casings : Shell Case Fabricators (SCF) designs and builds shell casings that enclose electronic products such as calculators, cell phones, modems.

What do the constitutive equations tell you about : Solid Mechanics - construct a set of equations from which you might obtain valid estimates for the forces in the two supporting members, BD and CD

Evaluate ubers legal exposure for the conduct of its drivers : While creating a new type of entrepreneurship for individuals, it raises a host of new legal questions for companies around the law of agency.

Discuss descriptive phenomenological psychology method : Quantitative research methods in psychology are based on the research methods used in the natural sciences disciplines to produce empirical research.

Identify issues in the current business environment : Identify issues in the current business environment that affect the demand and supply of engineering and technical personnel.

What do nrms have that more traditional religions : Since the 1960s in the United States, we have seen a proliferation of new religious movements (NRMs). These groups are often syncretic, blending elements.

User Account

All Pages