What are the arrow-debreu commodities in this economy

Reference no: EM131136039

Consider an economy consisting of H households each with a utility function at time t = 0 given by

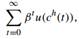
With β ∈ (0, 1), where ch(t) denotes the consumption of household h at time t. Suppose that u(0) = 0. The economy starts with an endowment of y > 0 units of the final good and has access to no production technology. This endowment can be saved without depreciating or gaining interest rate between periods.

(a) What are the Arrow-Debreu commodities in this economy?

(b) Characterize the set of Pareto optimal allocations of this economy.

(d) Consider an allocation of y units to the households, .

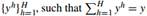
Given this allocation, find the unique competitive equilibrium price vector and the corresponding consumption allocations.

(e) Are all competitive equilibria Pareto optimal?

(f ) Derive a redistribution scheme for decentralizing the entire set of Pareto optimal allocations.

Reference no: EM131136039

Questions Cloud

How hr department aligns with organization strategic plan : Include/Explain at least four (4) different functions that are in the structure of a Human Resources Department and a brief description of each. (must use text here) Explain how the Human Resource department aligns with the organization's strateg..

Pros and cons of straight ticket voting election policy : What are the pros and cons of straight ticket voting election policy in Texas

What considerations needs support organizing a jad session : Why is it a team approach preferable to obtain system requirements - What considerations and project needs support organizing a JAD session?

Discuss the value of serving on a jury : Read over the "Participate!" section at the end of Chapter 14. Discuss the value of serving on a jury and the importance of citizen participation in the judicial process

What are the arrow-debreu commodities in this economy : Derive a redistribution scheme for decentralizing the entire set of Pareto optimal allocations.

Why are fish in the ocean an example of a re source : Why are fish in the ocean an example of a re- source that suffers from the problem of the commons but cattle grazing in a farmer's pasture do not suffer from the problem of the commons?

Describes the event and explains its background. : Current event must be pertinent to one or more of the fourteen topics covered in this course and has political dimensions. Write a summary analysis that describes the event and explains its background

Project integration managements final process : Project integration management’s final process is to close the project. Which of the following is a reason for this process? a. To indicate the project has ended b. To release all resources from the project c. To celebrate the conclusion of the proje..

Determine the quick ratio for the construction company : Determine the quick ratio for the construction company in Figures 6-1 and 6-2. What insight does this give you into the company's financial operations?

User Account

All Pages