Reference no: EM13371603
The aim of this demonstration is to use measurements of the load required to propagate a crack (tear) in packaging tape to determine its fracture toughness.
We will also observe what happens when a load is applied to a material with a sub-critical sized crack.
Governing equations:
From lecture:
Cracks propagate when the stress intensity factor exceeds a critical value - the critical value is the fracture toughness K1c
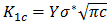
whereσ* is the tensile stress at which the crack propagates
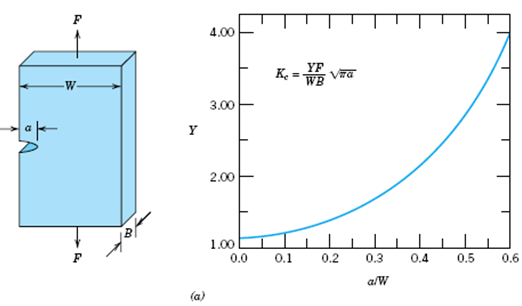
Questions
1. Use scissors to put a crack (cut) in a strip of the packaging tape (perpendicular to the direction of loading). Carefully apply weights to the bottom of the tape strip until the crack fast-propagates through the rest of its width. Repeat this at least 7 times with different sized cracks (cuts). Generate a table of crack length measurements and fracture load.
2. Convert load to stress and plot the data (taking into account the governing equation above) so that a fitted line can assist you in determining the fracture toughness. i.e. do not just plot stress against crack size. What is the fracture toughness, and hence, what material do you believe this packaging tape is?
3. For three small crack sizes (that are within the range of those in the table from question 1), place a vertical slit in the tape just in front of the crack tip and repeat the loading experiment. Plot these three points onto your graph obtained in question 2. Do the slits increase or decrease the stress required for fracture. Explain why
4. Describe the fracture behaviour of the insulation tape loaded with a small crack. Explain why it is different to that of the packaging tape.
5. The concept of yield-before-break is an important one used in many applications. In this concept, the critical crack size cannot be reached since the material will yield before a high enough stress can occur to propagate the crack. Determine the material index for this condition (this can bee done by setting the critical stress required to propagate the crack equal to σy). Use the CES software to select the best yield-before-break material for a pressure vessel that has an outer diameter of 0.5 m, must contain a pressure of 4 MPa and cannot have a thickness greater than 5 mm.