Problem 1 consider the following two-player static

Reference no: EM13375985

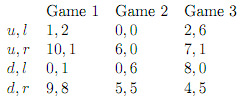
Problem 1: Consider the following two-player static simultaneous-move game with perfect information. Player 1 chooses either ëupí(u) or ëdowní (d): Player 2 chooses either ërightí (r) or ëleftí (l). The payo§s for both players of three versions of this simple game are presented in the following table, where the first entry corresponds to player 1ís payo§ and the second entry corresponds to player 2ís payo§. For each of the three games, express the payo§ information matrix in the normal form as presented in class and find all pure strategies Nash Equilibria in each version of the three games. Show all your work.

1203_Determine the equilibrium price and each firmís profit.png

Problem 2:

Consider the following game played by two firms: Firm A and firm B. Each firm can either produce a low output (L) or a high output (H). The low output is a two player collusion output. The high output is the competition output. The payo§s are given in the following payo§ matrix

865_Determine the equilibrium price and each firmís profit1.png

(a) Check for strictly dominant strategies for both players. Show your work.

(b) Find all Nash equilibria in pure strategies.

(d) Say both firms want to make the collusion outcome in (c) sustainable. How can they introduce a credible threat to achieve this?

(e) If both firms fail to maintain a stable collusion. What is the maximum benefit from cheating that can be realized?

Problem 3:
British Airways and Korean Air Fined Millions for Fixing Prices. Associated Press, August 24, 2007

WASHINGTON, Aug. 23 (AP) ó Two major airlines were fined $300 million each Thurs- day after admitting that they had conspired to fix prices on international áights and agreeing

to help prosecutors investigate other airlines. The airlines, ó British Airways, Britainís largest airline, and Korean Air, South Koreaís national carrier ó pleaded guilty to antitrust conspiracy charges. They acknowledged colluding with rivals over cargo rates and fuel surcharges, which had been added to fares in response to rising oil prices. That meant higher costs for international shippers and passengers. Judge John D. Bates of Federal District Court in Washington sad the case "involved considerable commerce and reáected long-term and widespread conduct involving major airlines and players." "Any anticompetitive behavior is to be condemned at British Airways or at other companies. It will not be tolerated and we remain vigilant in this respect," British Airwaysíchief executive, Willie Walsh, said in a statement released after the court proceedings. Korean Air released a statement saying it was "committed to antitrust compliance" and was taking steps to make sure the conduct was never repeated. Neither company addressed the extent of its cooperation or how widespread the practice was in the industry.

(a) Set up the matrix of profits depending on the pricing policy of each airliner. The possible profit levels under di§erent pricing policies are $400, $600, $800 and $1,000 (all values are in millions).

(b) Show and explain the outcome of this game that the two airliners were trying to achieve. How easy it is for this outcome to be reached?

(d) Is the $300 million penalty su¢ cient to deter future price-fixing, assuming that the profit matrix stays constant? Explain

Problem 4:

Find the NE of the following game using the iterative elimination of strictly dominated strategies that was discussed in class. Show your work.

107_Determine the equilibrium price and each firmís profit2.png

Problem 5:

Consider two firms, 1 and 2, each producing an identical good simultaneously, this good has a market demand given by the (inverse) demand function p = 10 Q; where p is the price and Q = q1+q2 is market quantity. qi represents the amount produced by firm i: Both firms have an identical cost structures given by Ci = yi; where = 1 and there is no fixed cost.

1. Solve for the Cournot-Nash equilibrium. Express your answer graphically by drawing each firmís reaction function and showing the equilibrium point.

2. Determine the equilibrium price and each firmís profit.

3. Solve for the monopoly outcome, i.e., find the monopoly quantity, the monopoly price and profit.

4. Design and explain a strategy that makes the outcome found in (3) sustainable.

Reference no: EM13375985

Questions Cloud

Bob and bette rhymes with jetty each have cobb-douglas : bob and bette rhymes with jetty each have cobb-douglas preferences for cheese c and peanut butter p each of which will

Honda uses flexible plans in the manufacturing of its cars : honda uses flexible plans in the manufacturing of its cars. discuss whether this method of production results in

Question 1 a suppose the income elasticity of demand for : question 1 a suppose the income elasticity of demand for furniture is 3 and the income elasticity for doctors services

The products profits per unit output are 50 per gallon for : the products profits per unit output are .50 per gallon for gasoline and .75 per square foot for fiber.nbsp then the

Problem 1 consider the following two-player static : problem 1 consider the following two-player static simultaneous-move game with perfect information. player 1 chooses

In november 1990 chrysler corporation announced plans to : in november 1990 chrysler corporation announced plans to initiate three-shift or nearly continuous 21-hours-per-day

A country has had a steady value for its floating exchange : a country has had a steady value for its floating exchange rate stated inversely as the domestic currency price of

Problem 1like supermarkets full-service department stores : problem 1like supermarkets full-service department stores like macys are generally in decline. what factors might these

A selfless person approaches jones and smith with a 100 and : a selfless person approaches jones and smith with a 100 and offers to sell it to the highest bidder but both the

User Account

All Pages