Monte carlo risk analysis model

Reference no: EM133147410

Question 1. Convert this influence diagram into a decision tree. All Decisions and Risks are binary (have only two states, e.g., black/white, up/down, hot/cold)

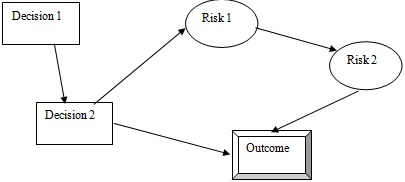
736_Decision tree.jpg

a) Of the five connecting arrows, how many could show relevance not merely timing? Why?
b) Can there be more than one correct representation of the decision tree corresponding to this influence diagram? If no, why not. If yes, about how many different "correct" trees are there?
c) What does the arrow between Risk 1 and Risk 2 signify?
d) Is Outcome a function of Risk 1, Risk 2 and Decision 2 or just Risk 2 and Decision 2? Why

Question 2 A project's uncertainty was evaluated using a Monte Carlo risk analysis model. The following results were obtained:

Original estimated project cost = $14,500,000
Evaluated (mean) project cost = $23,800,000
Evaluated maximum cost = $33,000,000
Evaluated minimum cost = $12,800,000
Evaluated standard deviation = $3,300,000
The shape of the distribution created from the Monte Carlo is approximately Normal (Gausian).

When the project was completed the True (actual) final project cost = $27,300,000

Was the uncertainty/risk evaluation good (accurate, useful) for the project team? Note your assumptions and provide numerical justification for your answer to this question.

Question 3. A highway construction contractor has developed a base estimate for a project of $18,500,000. Additionally, they completed a qualitative risk analysis in preparation for their bid based on the following information.

Risk Register

Risk Description	Probability of Occurrence	Cost Impact
Delay due to extreme weather	Medium	Very Low
Delay in obtaining permit	Low	Low
Subcontractor default	Very Low	High
Failure to pass quality control test	Medium	Low
Delay in material delivery	High	Very Low

Cost Impact Probability of Occurrence

Description	% of Base Cost	Description	Probability
Very Low	0-1%	Very Low	0-5%
Low	1-2%	Low	5-10%
Medium	2-5%	Medium	10-30%
High	5-10%	High	30-70%
Very High	10-20%	Very High	70-100%

a. Given only the information above, rank the risks in order of importance.
b. Given only the information above and using the mean value method, what contingency should the contractor include in its bid?
c. Please provide three specific reasons why this analysis of contingency might be insufficient or incorrect.

Reference no: EM133147410

Questions Cloud

Describe three laws and regulatory organizations : Identify and describe three laws and/or regulatory organizations that are working to reduce corruption worldwide. Be specific.

Corruption perceptions index : Think back in the country research tools we have introduced in D270. Which of the tools do you think is most important for an MNE that is deciding whether or no

How much should the city report as expenditures : How much should the city report as expenditures in the Debt Service Fund in its year-end fund level financial statements on December 31, 2023

Products in development that pfizer is currently working : Are there any reported products in development that Pfizer is currently working on that no one has a product yet?

Monte carlo risk analysis model : Of the five connecting arrows, how many could show relevance not merely timing and What does the arrow between Risk 1 and Risk 2 signify

Create lasting competitive advantage : What are the company's most important resources and why? How do the company's most important resources and capabilities create lasting competitive advantage?

Explain how export subsidy is theoretically meant to work : Explain how an export subsidy is theoretically meant to work. Think of the application to "infant industry".

Design plan to increase satisfaction : Estimate the relative importance of performance factors your organization's customers evaluate and design a plan to increase satisfaction

Develop market positioning strategies : Select a product of a chosen organisation that has went through the four stages of product life cycles; introduction, growth, maturity and decline.

User Account

All Pages