Reference no: EM13517697

Track Inspection Planning

Dr. Konur has recently completed a project for Missouri Department of Transportation, which was for optimizing the track inspection planning on the Missouri railroad network. In this question, you are asked to formulate a simpler version of the track inspection planning problem.

In particular, suppose that there 5 rail tracks that you can inspect. Each track has different inspection importance and each track has different inspection times. The table below gives the importance level and inspection time for each track.

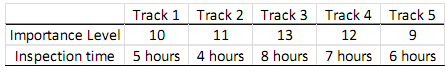
As the inspection planner, you want to determine which tracks to inspect such that you maximize the total importance level of the inspections. However, you have one day, i.e., 24 hours available for inspections. That is, total inspection time cannot exceed 24 hours.

a)  Formulate a binary linear programming model for the above inspection planning problem by defining you decision variables and writing the objective and objective function and the constraints in terms of your decision variables. Combine everything to get the final model.

b) Mathematically formulate the following restrictions as constraints independent of each other and the constraints in part a. You should formulate a single constraint for each part.

a. You have to inspect at least 3 tracks.
b. If you inspect track 1, then you have to inspect track 2.
c. You can either inspect track 3 or track 4, but not both.
d. If you inspect both track 1 and track 2, then you have to inspect track 4.
e. You cannot inspect track 3 unless you inspect track 4.
f. You cannot inspect track 1 unless you inspect track 3; and, you cannot inspect track 3 unless you inspect track 1.

c) Mathematically formulate the following restrictions as constraints independent of each other and independent of the constraints in parts a and b (you might need more than one constraints in some parts or you might need to define new decision variables in some parts).

a. If you inspect track 3, you can inspect at most 2 other tracks.
b. If inspect 1 or less tracks, then track 4 cannot be inspected. That is, track 4 cannot be the only inspected track.
c. If you inspect 1 or more tracks, you have to inspect at least 2 tracks.