How much variation is there in the routine analysis

Reference no: EM131197188

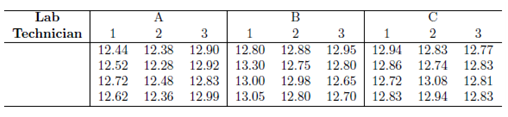
1. How much variation is there in the routine analysis of fibre content of soya cotton cakes? The data are presented by the lab that did the test and the technician within the lab that did the analysis.

(Data from Clarke and Kempson)

To perform an appropriate analysis, you will need 3 columns, one for the responses, one indicating the lab and one indicating the technician. When specifying your model, remember that technicians are nested within labs (so you could number the technicians from 1 to 9 if you wanted). Write down the two null hypotheses. Check whether or not the assumption of normality is justified. Give a 2 or 3 sentence summary of your conclusions.

2. Commercial respirators include filters to protect against particulate matter. There are two manufacturers of such filters and three filters were selected at random from each manufacturer. Each filter was tested three times. \The measurements are the percent penetration by a standard type of aerosol." (from Kuehl, including data)

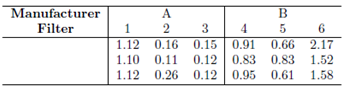
(a) Using these data , write down the appropriate linear model.

(b) Compute the analysis of variance table, including the expected mean squares.

(d) As the assumption of normality is not satisfied, replace the responses by their ranks and repeat

the analysis on the ranks. Have your conclusions changed?

(e) Write a 1 or 2 sentence summary of your conclusions.

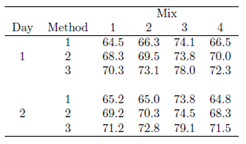
3. Montgomery (2007) describes an experiment to study pigment dispersion in paint. Four mixes of a pigment are used. Each day all four mixes are prepared and then applied to three panels with each panel receiving paint by one of three application methods (brush, roller, spray gun). The experiment is repeated on a second day. Perform an appropriate analysis and comment. (Hint: consider the paper tensile strength example.)

Reference no: EM131197188

Questions Cloud

What is the probability that both will satisfy specification : A certain application specifies capacitors with resistances between 900 and 1,000 megohms.

Force on the negative charge : A 9.5 μC charge is at x1 = 14 cm , y1 = 7.5 cm and a -5.0 μC charge is at x2 = 4.5 cm , y2 = 11 cm. Find the force on the negative charge. Include Fx and Fy measured in N.

Minimum charge required to pick up the tissue paper : You hold a ruler that has a charge on its tip 2 cm above a small piece of tissue paper to see if it can be picked up. The ruler has -14 µC of charge. The tissue has 1 g of mass. What is the minimum charge required to pick up the tissue paper?

Confined to be within a nucleus : Consider a proton moving in 3 dimensions, whose motion is confined to be within a nucleus (a sphere of radius 2e-15 m) and whose momentum must have magnitude less than p0= 3e-19 kgm/s.

How much variation is there in the routine analysis : How much variation is there in the routine analysis of fibre content of soya cotton cakes? The data are presented by the lab that did the test and the technician within the lab that did the analysis.

Determine the magnitude and direction of the electric field : Determine the magnitude and direction of the electric field at the location of charge q. (Use k_e for the Coulomb constant and q and a as necessary.)

Write about work-family balance : Write about Work-Family Balance. From Work-Family Conflict To Work-Family Enrichment: Competing Negative and Positive Views

What are the essential functions of the position : What are the duties and job responsibilities associated with the position held by the individual you interviewed? What are the types of knowledge, skills, and abilities that are needed to successfully accomplish the job responsibilities?

What fraction of these printers will fail before 1,000 hours : What should be the guarantee time for these printers if the manufacturer wants only 5% to fail within the guarantee period?

User Account

All Pages