Reference no: EM132736821

QUESTION 1

You run the linear regression using one variable using SPSS statistics, which provides the following output:

Model            Coefficient        Std, Error
1 - Constant       80                  580
SystolicBP          1.82               .137

What is the linear regression equation for this model?
1.y = 80* SystolicBP + 1.82
2.y= 580 * SystolicBP + 80
3. y = 1.82 * SystolicBP + 80
4.y= .137*SystolicBP + 80

QUESTION 2

If University GPA' = (0.675) (High School GPA) + 1.097  is the linear regression for the university GPA, what is the interpretation for the coeficient 1.097.

1. Because a value of 0 for the (High School GPA) is not applicable in this model the y-interceptor 1.097 has not a valid meaning in this case.
2.1.097 is the average error the model would make when (High School GPA)=O
3. For a student with (High School GPA)=0 we can expect a value of 1.097 GPA when he(r) joins the university.
4. The y-interceptor of 1.097 does not have any special meaning.

QUESTION 3
Among the possible lines that can be used as estimation model which line do we pick?
1.There is only one line that approximates the observed values and therefore optimum by definition.
2. We pick the line that minimize the sum of squared error function.
3. We pick the line that minimize the quantity:
∑(y'-y)
With y' the estimated value of the regression line.
4.We pick the line that maximizes SSE.

QUESTION 4

What is the hypothesis testing we conduct on the linear regression model in one variable as the one below?
y=β0+βx+ ∈
01.
H0: β₀ =0 No relationship between x and y.
Ha: β₀ ≠0 Linear relationship between x and y.
02.
H0 β₀≠ 0 Linear relationship between x and y.
Ha: β₀ = 0 No relationship between x and y.
3.
H0: β₀ = 0 No relationship between x and y.
Ha: β₀ ≠ 0 Linear relationship between x and y.

QUESTION 5
You generate the scattered plot of TestScore and HoursStudied as shown in the picture:

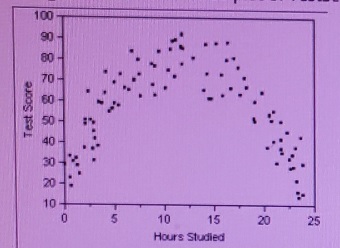
You decide to go ahead and generate a linear regression to predict the test score outcome.
1. You can create a linear regression model only for students that study up to 8 hours, it would be a mistake to assume the same
relationship for values >8.
2. No you cannot build a linear regression model since the relationship between the two variables is clearly non-linear.
3. You can build the linear regression model: it does not really matter the type of relationship shown by the scattered plot.
4. You can build the linear regression, knowing the model will have a large estimation error. However, since you know the curviinear
relationship you can adjust for the error.

QUESTION 6

What is the test statistics used to verify the fit for the independent variable 3 in the linear regression

Y=β₀+β_x + ∈
1. The test statistics is t=β/S_b with sb the standard error of the coefficient β.
2. The test statistics is t=β₀/S_b with sly the standard error of the coefficient β₀.
3.The test statistics SSR/SST with SSR equal to the Sum of Square Regression and SST equal to the Total' Sum of Square
4. The test statistics S=√MSE.