Reference no: EM131023046

Homework 2-

1. Absolute Advantage and Comparative Advantage:

The following is From Elements of Political Economy, Chapter III, Section IV, by John Stuart Mill. First published 1821:

If two countries can both of them produce two commodities, corn, for example, and cloth, but not both commodities, with the same comparative facility, the two countries will find their advantage in confining themselves, each to one of the commodities, bartering for the other. If one of the countries can produce one of the commodities with peculiar advantages, and the other with peculiar advantages, the motive is immediately apparent which should induce each to confine itself to the commodity which it has peculiar advantages for producing. But the motive may no less exist, where one of the two countries has facilities superior to the other in producing both commodities.

By superior facilities, I mean, the power of producing the same effect with less labour. The conclusion, too, will be the same, whether we suppose the labour to be more or less highly paid. Suppose that Poland can produce corn and cloth with less labour than England, it will not follow that it may not be the interest of Poland to import one of the commodities from England. [...] if, at the same time that the quantity of cloth, which, in Poland, is produced with 100 days' labour, can be produced in England with 150 days' labour; the corn, which is produced in Poland with 100 days' labour, requires 200 days' labour in England; in that case, it will be the interest of Poland to import her cloth from England. The evidence of these propositions may thus be traced.

[...] while the cloth produced with 100 days' labour in Poland was produced with 150 days' labour in England, the corn which was produced in Poland with 100 days' labour could not be produced in England with less than 200 days' labour; an adequate motive to exchange would immediately arise. With a quantity of cloth which England produced with 150 days' labour, she would be able to purchase as much corn in Poland as was there produced with 100 days' labour; but the quantity, which was there produced with 100 days' labour, would be as great as the quantity produced in England with 200 days' labour. If the exchange, however, was made in this manner, the whole of the advantage would be on the part of England; and Poland would gain nothing, paying as much for the cloth she received from England, as the cost of producing it for herself.

But the power of Poland would be reciprocal. With a quantity of corn which cost her 100 days' labour, equal to the quantity produced in England by 200 days' labour, she could in the supposed case purchase, in England, the produce of 200 days' labour in cloth. The produce of 150 days' labour in England in the article of cloth would be equal to the produce of 100 days' labour in Poland. If, with the produce of 100 days' labour, she could purchase, not the produce of 150, but the produce of 200, she also would obtain the whole of the advantage, and England would purchase corn, which she could produce by 200 days' labour, with the product of as many days' labour in other commodities.

a. Assume "peculiar advantage" stands for "absolute advantage" in our up to date terminology. What does the assertion in the last sentence of the first paragraph refer to?

b. Plot the PPF curve of both Poland and England according to the example in the text. In your graph measure corn on the horizontal axis and cloth on the vertical axis. Assume each of the countries is endowed with 600 days of labor, and that the PPF of the two countries are linear.

c. For both goods describe which country has the comparative advantage and the absolute advantage.

d. Use the text to describe what are the ratios of corn to cloth, in which Poland and England can trade while maintaining beneficial trade for both countries.

2. Math Review - Linear Equations:

Linear relations between two variables can be expressed in few ways. Let us summarize these ways in the following definitions:

Graphic - using a line plotted on a specified axis system.

Algebraic - using an equation that relates the two variables and contains both slope and the intercept as parameters.

Numeric - specifying at least two points (x1, y1) and (x2, y2) that lie on the line in the graphical description, or that satisfy the algebraic relation.

In the following table there are different descriptions of lines. Complete the missing information in the table. The first row is already completed for you.

 

Algebraic	Numeric	Graphic
y= 3x+5	(x1, y1)= (1, 8) (x2, y2)= (4, 17)
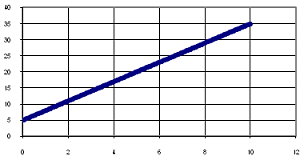
b. ?	(x1, y1)= (0 ,5) (x2, y2)= (1 ,2)
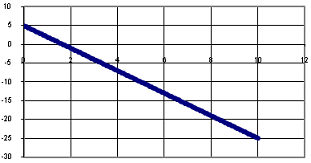
c.       x= - (1/3)y+(5/3)	?	?
d. y = 2x-?	(x1, y1)=(1,-5)	?
e. ?	?
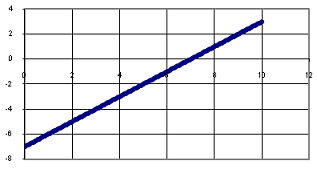
f. ?	(x2, y2)= (0, 0)
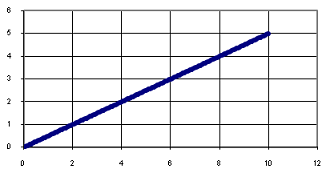
g.       y= ?x-2	(x1, y1)=(1.5,1)	?
h.      (y/3x)= ?	(x2, y2)=(8,24)	?

3. Supply, Demand and Taxes:

The government wishes to raise money through an excise tax levied on producers of cigarettes. The purpose of the tax is to buy medical equipment to fight health problems that arise as a result of smoking. The more people smoke, the more health problems there are, and in particular the total cost of handling the health problems is C=8*Q, where Q is the quantity of cigarettes consumed in the market and C is the total cost to society of smoking. The demand and supply curves in the cigarette market (before any tax is introduced to the economy) are the following:

Demand: P = 80 -5*Q

Supply: P = 3*Q

a. What is the equilibrium price and quantity in this market before any government intervention?

b. After the tax is introduced what happens to the supply and demand curves? Do any of the curves shift? If so, in which direction?

c. What is the equilibrium price and quantity in this market after the government introduces the tax? [Hint: If the total cost is 8*Q, then the cost per unit would be equal to 8*Q/Q = 8.]

d. Calculate the consumer surplus, producer surplus, the tax revenue to the government, and the dead weight loss due to the taxation of cigarettes. (In calculating DWL note that before taxation smoking has a negative externality as it induces health costs). Add a graphical illustration of these quantities.

Now assume that enough medical equipment has been purchased and the government wishes to launch a campaign against smoking. The more expansive the campaign the more effect it has on smokers: as the campaign gets more expansive the less smoking will occur. In particular, a campaign with cost d, would alter the demand in the following way:

D': P = (80-d) - 5*Q

The government still wishes to fund the campaign through the same excise tax as before. That is, the money collected through the tax is used to fund the campaign, and nothing else. In effect, d, the amount of money spent on the campaign, must equal the amount of money collected through the tax, C. Assume all money collected through the tax is used to fund the campaign to reduce smoking. 

e. After the tax is introduced what happens to the supply and demand curves? Provide equations for the new supply curve and the new demand curve. Do any of the curves shift? If so, in which direction? [Hint: think about whether the demand or supply curves shift in a parallel fashion or whether they pivot around their y-intercept.]

f. What is the price and quantity in equilibrium in this market?

g. From your work in part (f), what is the value of d?

h. Given your work in (e) and (f), what are the values for consumer surplus, producer surplus and government tax revenue given the imposition of the excise tax and the campaign to discourage smoking? [Hint: Base your calculations on using the new demand curve and the new supply curve.] Add a graphical illustration of these quantities. Can you say something about where d lies in your graph?

4. International Trade - Quota, Tariff and Dead Weight Loss:

Consider a small economy that produces a good in a negligible amount relative to the world production, and where the market for this good can be characterized by the following equations:

Domestic Demand: P= 120-Q

Domestic Supply:  P= 2Q

World price of good: P= 20

a. Find the price and quantity of this good when it is produced in the small economy with the market closed to world trade.

b. Find the price, quantity produced locally and quantity consumed when the market is opened to world trade. Identify the level of imports or exports.

c. Now assume the market is open to trade and the government introduces a $10 tariff on importing the good. Find the new equilibrium price, the quantities produced and consumed locally; and calculate the consumer surplus, producer surplus, tariff revenue and dead weight loss from the imposition of this tariff. Illustrate your answer in a graph.

d. Finally, the government changes its taxation policy. It sells a permit to import 9 units of the good to a single importer through an auction. Assume the import market is competitive. What will be the price of this permit? Explain your answer. Add a graphical illustration if helpful.