Reference no: EM13919763
1. Consider the complex sequence
ejω0n, 0 ≤ n ≤ N - 1.
x[n] =
0, otherwise.
(a) Find the Fourier transform X(eiω) of x[n].
(b) Find the N-point DFT X [k] of the finite-length sequence x[n).
(c) Find the DFT of x[n] for the case ω0 = 2πk0/N, where k0 is an integer.
2. Suppose we have two four-point sequences x[n] and h[n] as follows:
x[n] = cos (πn/2), n = 0, 1, 2, 3.
h[n] = 2n, n =0, 1, 2, 3.
(a) Calculate the four-point DFT X [k].
(b) Calculate the four-point DFT H[k].
(c) Calculate y[n] = x[n]4h[n] by doing the circular convolution directly.
(d) Calculate y[n] of pan (c) by multiplying the DFTs of x[n] and h[n) and performing an inverse DFT.
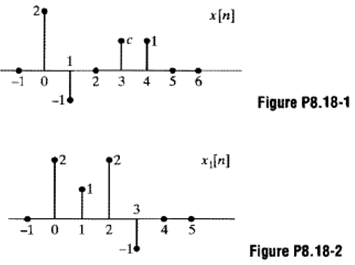
3. Figure P8.18-1 shows a sequence x[n] for which the value of x[3] is an unknown constant c. The sample with amplitude c is not necessarily drawn to scale. Let X1[k] = X [k]ej2π3k/5, where X [k] is the five-point DFT of x[n]. The sequence x1[n] plotted in Figure P8.18-2 is the inverse DFT of X1[k]. What is the value of c?
4. Consider the signal x[n] = δ[n - 4] + 2δ[n - 5] + δ[n - 6].
(a) Find X(ejω) the discrete-time Fourier transform of x[n]. Write expressions for the magnitude and phase of X (ejω), and sketch these functions.
(b) Find all values of N for which the N-point DFT is a set of real numbers.
(c) Can you find a three-point causal signal x1[n] (i.e., x1[n] = 0 for n < 0 and n > 2) for which the three-point DFT of x1[n] is:
X1[k] = |X[k]| k = 0, 1, 2
where X [k] is the three-point DFT of x[n]?
5. An FIR filter has a 10-point impulse response, i.e.,
H[n] = 0 for n < 0 and for n > 9.
Given that the 10-point DFT of h[n] is given by
H[k] = 1/5δ[k - 1] + 1/3δ[k - 7], find H(eJω), the DTFT of h[n].
6. Suppose that x1[n] and x2[n] are two finite-length sequences of length N, i.e.. x1[n] = x2[n] = 0 outside 0 ≤ n ≤ N -1. Denote the z-transform of x1[n] by X1(z), and denote the N-point DFT of x2[n] by X2[k]. The two transforms X1(z) and X2[k] are related by:
X2[k] = X1(z)|z = 1/2e-j(2πk/N). , k = 0, 1, . . . , N - 1
Determine the relationship between x1[n] and x2(n].
|
How the market would set the yield to maturity
: Blue Crab, Inc. plans to issue new bonds, but is uncertain how the market would set the yield to maturity. The bonds would be 29-year to maturity, carry a 8.02 percent annual coupon, and have a $1,000 par value. Blue Crab, Inc. has determined that th..
|
|
More complex problem, develop a complete solution
: Your algorithm will keep track of a customer's purchases at the local fireworks stand. Customers will not know exactly how many items they will purchase, so using a For loop on this lab is not allowed. Let's keep the rules simple.
|
|
When a doctor proposes a new surgical procedure
: When a doctor proposes a new surgical procedure, how do you recommend the SKU for a new custom pack be entered into the hospital's supply-chain system?
|
|
Two WACC components-Debt and Equity
: Assume that Marriott uses only two WACC components – Debt and Equity (common stock). Calculate the WACC for each of the three Marriott divisions (lodging, contract services, and restaurants). Be sure to document and explain the reasons for any assump..
|
|
Find the fourier transform
: Find the Fourier transform X(eiω) of x[n]. Find the N-point DFT X [k] of the finite-length sequence x[n). Find the DFT of x[n] for the case ω0 = 2πk0/N, where k0 is an integer
|
|
Describe the organization current hiring needs
: Evaluate the cultural issues that need to be addressed. Analyze the ethical considerations that apply. Describe the organization's current hiring needs and projected hiring needs for the coming year. Develop a recruitment plan for fulfilling the comp..
|
|
Record the events in general ledger accounts
: Record the events in general ledger accounts under an accounting equation.
|
|
What is the current price of value bond maturing
: What is the approximate yield to maturity for a $1000 par value bond selling for $925 that matures in 8 years and pays a 10 percent coupon that is paid semiannually? What is the current price of a $1000 par value bond maturing in 12 years with a coup..
|
|
Assignment on financial statement analysis
: Select one (1) of the following publically traded health care organizations: Universal Health Services (NYSE: UHS) or Health Management Associates (NYSE: HMA).
|