Reference no: EM131139817

1. For each of the following functions, find ∂f/∂x, ∂f/∂y and df/dx-

a) f (x, y) = 24x^2/3y^1/3.

b) f(x, y) = In(x²)+ 4√y

2. Consider the function

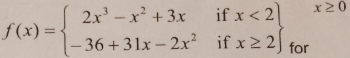
(Note- If you present any answers as decimal approximations, round to three decimal places)

a) Explain why f(x) is continuous and differentiable.

b) Graph the function in the xy plane where both x and y are non-negative. Find the positive values of x over which the function is increasing and decreasing. What value of x maximizes the function? (Hint: It is possible that doing part (c) first may make this part easier)

c) Calculate the derivative of the function and explain why the sign of the derivative makes sense given your answers to part (b).

d) In your graph in (b), what value(s) of x minimize(s) the function for the domain and range given in (b)? Is df/dx for that (these) values of x? Why or why not?

e) Explain why = 2 is a point of inflection for f(x).

3. If g(x) = √(x+3) and f(x) = 2x³ - 1 then calculate d/dx(g(x)/f(x)).

4. Find the numerical value of the area between the y-axis, the line y = 3 and the function y = x^1/2.