Reference no: EM132047

Question 1. A mild steel bar 40 mm diameter and 100 mm long is subjected to a tensile force along its axis.

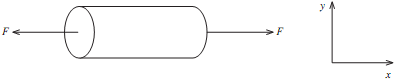
Young's modulus of elasticity for mild steel = 200 GN m^-2. Poisson's ratio is 0.3.

Evaluate the force (F) required to reduce the diameter to 39.99 mm. Use the x-y coordinate system as shown above.

Question 2. A tubular column supports a mass as given in the diagram below. A strain gauge is placed transversely on the outside diameter of the bar to act as a load measuring device.

The column has an outside diameter of 50 mm and an inside diameter of 40 mm. The modulus of elasticity of the tube material is 250 GN m^-2 and the poison's ratio is 0.33.

(a) Show a graph of the expected strain against the applied load, for a load range from 0 to 500 kg. Make load the horizontal axis on the graph and strain the vertical axis.

(b) Show with the aid of a diagram how the orientation of the strain gauge could be changed to give a greater strain for the same load.

(c) Show a graph of strain against applied load when the strain gauge is in the new position.

Ignore the weight of the column in all calculations.Use the x-y coordinate system as shown below

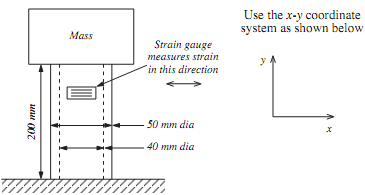
(d) Evaluate the change in:

(i) the outside diameter (Do)
(ii) the inside diameter (Di)
(iii) the column length (l)

under the load. Indicate whether the dimension has increased or decreased.

(e) With the aid of sketches show how four strain gauges will be used in conjunction with a Wheatstone bridge to give maximum sensitivity of the load measurement system. Show clearly the orientation of the strain gauges on the tubular column and the positioning of these gauges on the Wheatstone bridge.

Question 3. The simply supported beam given in FIGURE 1 is 10 m long with E = 200 × 10⁹ Pa and I = 150 × 10^-6 m⁴.

For the beam in FIGURE :

(a) (i) Evaluate the position and magnitude of the maximum bending moment.

(ii) Plot a graph of the deflection along the length of the beam (determine the deflection at 1 m intervals).

(b) What I value for the beam would be needed to halve the maximum deflection of the beam ?

(c) The evaluation for the maximum bending moment is to be verified experimentally using a strain gauge bonded to the outer surface of the beam, at the point where the maximum bending moment occurs.

Derive an equation which could be used to evaluate the bending moment from the measured strain value. State the meaning of all symbols used in your equation.

Question 4. 20 m³ of gas at a pressure of 25 bar is to be stored in a cylindrical pressure vessel 2 m long. Given the following information :

The yield strength of the vessel material is 14,000 psi If a factor of safety of 5 is to be used, evaluate:

(a) Whether the vessel could be treated as a thin or thick cylinder.

(b) The wall thickness needed for the pressure vessel.

Question 5. A pulley 150 mm diameter is driven directly by an electric motor at 250 revs min-1. A V-belt is used to transmit power from this pulley to a second pulley 400 mm diameter against a load of 200 Nm.  The distance between the centre of the pulleys is 600 mm, the included angle of the pulley groove = 40°, the coefficient of friction between the belt and pulley is 0.4 and the ultimate strength of the belt is 8 kN. 

(a) Determine the actual power transmitted to the second pulley.

(b) Determine the power which can be transmitted if the maximum tension in the belt is limited to half of the ultimate strength of the
belt.

(c) What could be the effect of the following factors on the maximum power which can be transmitted (give reasons for your answer):

(i) increasing the coefficient of friction
(ii) increasing the included angle of the pulley groove.

(d) What would be the effect on the following if the load torque is increased and the speed maintains constant (give reasons for your
answer):

(i) the tension in the tight side of the belt

(ii) the tension in the slack side of the belt

(iii) the power transmitted.

Question 6. A gear train is to be designed to transmit power from shaft 1 to shaft 2 with the given condition:

(i) Shaft 1 (driven by an electric motor) rotates at 100 revs min-1.

(ii) Shaft 2 is to rotate at 400 revs min-1 in the opposite direction to shaft 1 against a load of 200 Nm.

(iii) The centre of shaft 1 is 300 mm from shaft 2.

(iv) The minimum number of teeth on any gear is 15, all gears must have a multiple of 5 teeth and have a module of 2 mm.

(v) The maximum number of gears permissible is 4 gears and the diameter of the maximum gear must be minimised.

(vi) The centres of the gears should lie on a line which is as close to straight as possible.

(vii)All shafts have a frictional resistance of 5 Nm.

Carry out the following:

(a) Design a gear train which satisfies the given criteria. Use a sketch to explain your design, label the gears A, B, C, etc. from the driver to the driven gear, and state the number of teeth on each gear.

(b) Evaluate the input power required at shaft 1.

(c) Specify the efficiency of the gear train as a percentage.

(d) Evaluate an equation for the efficiency of the gear train in terms of the load (torque) on shaft 2 (all other factors remaining constant).

Question 7. (a) For the mechanism shown in FIGURE 1 determine for the angle θ = 45°:

(i) the velocity of the piston relative to the fixed point O (VBO)
(ii) the angular velocity of AB about point A (i.e. ωAB)
(iii) the acceleration of point B relative to A (aBA).

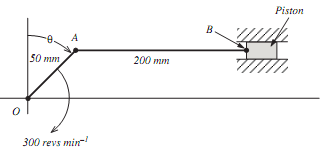
Note: Link AB is horizontal when θ = 45°

(b) Evaluate the value of the angle θ (measured from vertical) when:

(i) the velocity of point B = 0
(ii) the angular velocity of link AB a maximum.
State the angle θ anticlockwise from the horizontal position as shown.

(c) What is the maximum angular velocity of link AB?

Question 8. (a) A shaft 2 m long rotates at 1500 revs min-1 between bearings as shown in FIGURE. The bearings experience forces of 5 kN and 3 kN acting in the same plane as shown. A single mass is to be used to balance the shaft, so that the reactions are zero. The mass is to be placed at a radius of 200 mm from the shaft centre, 180° from the direction of the bearing reactions. Determine the size and position (a and b) of the mass to be used.

(b) The shaft in part (a) is to be balanced using two masses (m1 and m2) placed 0.5 m and 1.5 m from end A and 180° from the direction of the bearing reactions, each on radius arms 100 mm long. Determine the sizes of m₁ and m₂.

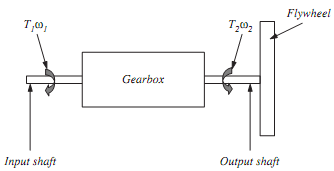
Question 9. A gearbox and flywheel are as shown in FIGURE 4. The output shaft rotates in the opposite direction to the input shaft at 5 times its speed.

The gearbox has an efficiency of 92%.

If the flywheel is solid, has a mass of 50 kg, a diameter of 1.5 m and is to accelerate from rest to 300 revs min-1 in 1 minute:

(a) Evaluate the torque required at input T1.

(b) Evaluate the magnitude and direction of the torque required to hold the gearbox stationary (holding torque Th). Show the direction of the holding torque applied to the shaft with the aid of a sketch.

(c) Draw a graph of the input power against time when taking the flywheel from rest to 300 revs min^-1.