Reference no: EM1380801

Problem 1: Estimating the Marginal Return to Schooling Suppose Carl's wage-schooling locus is given by the following schedule:

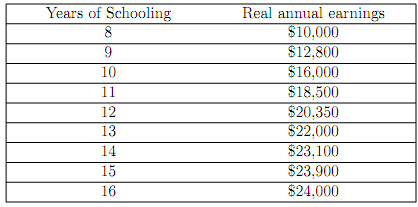
Suppose that the only cost of schooling is the opportunity cost of not working and earning money. Also assume that Carl's life is in?nitely long (this is a good approximation when the time horizon is long).

1. Derive the marginal rate of return schedule. (This is, calculate Carl's internal rate of return to each additional year of schooling).

2. When will Carl quit school if his discount rate is 4 percent? Why?

3. What will Carl's optimal schooling choice be if his discount rate is 12 percent? Why?

4. Suppose the government imposes an income tax of 20 percent. What is the e↵ect of this income tax on Carl's educational attainment? Why?

Problem 2: Schooling

Nancy is deciding on an optimal schooling strategy. She currently has zero years of schooling and knows she will live for 60 more years. In any given year she can either choose to add to her current level of schooling or she can work. If she decides to work, she will have an annual salary of √S, where S is her current level of schooling (measured in years of schooling). If she decides to go to school, she will not have any income during that year, but her schooling

level will increase by 1 year. Schooling is otherwise free. Nancy's discount rate is r =0.

Nancy's objective is to maximize lifetime income.

1. What is Nancy's lifetime income as a function of her level of schooling, S?

2. What is Nancy's lifetime income if she gets no schooling? What is it if she goes to school for all 60 remaining years of her life? In words, describe the "cost" to Nancy of choosing to attend school for 1 additional year.

3. Determine Nancy's optimal schooling level (hint: you can do this with calculus or by graphing her lifetime income and ?nding the level of schooling that maximizes that income).

4. During which part of Nancy's remaining life will she get her schooling?

Problem 3: Human Capital Investment

Suppose the economy consists of two types of individuals, type 1 and type 2. The two groups di↵er in their returns to schooling, S.The present value of earnings as a function of schooling is given by PV_i(S)= R_iS; i =1;2. Both types face the same education cost C(S)= . Each individual maximizes net income which is given by the present value of earnings minus the education cost.

1. Write up type i0s maximization problem over schooling.

2. It can be shown that the marginal bene?t of schooling for type i is R_i while the marginal cost is S/α for both types. Determine the optimal schooling level, S_i^*, as a function of α and R_i.
3. Determine the present value of earnings associated with type i's optimal schooling choice (that is, insert S_i^* into PV_i (S) for each type i =1;2).

4. Suppose α =100, R₁ = .05, and R₂ = .06. Determine (S_i^*; PV_i(S_i^*)) for each type i =1;2.

5. An econometrician will never observe R_i directly but is sometimes eager to estimate it based on observed combinations of schooling and present value of earnings in data. In our simple model, there will be two education levels in the economy, S₁^* and S₂^*,and there will be two associated earnings levels, PV₁* and PV₂* .

Based on these observations, the econometrician could estimate the returns to one additional year of schooling by calculating:

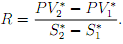
Determine this level (R).

6. How does this estimate compare to the actual returns to education for types 1 and 2, R₁ and R₂ ? Interpret the results.

Problem 4: GED's

A study shows that, for American high school dropouts, obtaining a General Equivalency Degree (GED) by part-time study after high school has very little payo↵. It also shows, however, that for immigrants who did not complete high school in their native countries, obtaining a GED has a relatively large payo↵. Can signaling theory be used to explain these results?