Reference no: EM13127964

Question 1  (Demand Elasticity )

Commuters in a medium-sized city can travel either by automobile or by bus. The demand for automobile travel has been estimated to be

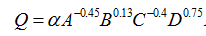 All exponents are constant elasticities (direct or cross).

where:

Q     =   automobile trips per hour (peak-hour)

a     =   constant

A     =   travel time by automobile (in minutes)

B     =   travel time by bus (in minutes)

C     =   average cost by automobile (in cents)

D     =   average cost by bus (fare, in cents)

 

(a)      The exponent of D in the above equation is +0.75.  What does this say about the effect of bus fare on the demand for auto travel?

(b)      Is the demand for auto with respect to auto travel-time elastic or inelastic?  Please explain.

(c)       Because of the congestion that is likely to occur on this corridor, the travel time by automobile would increase by 20%, and travel time by bus would increase by 10%.  At the same time, auto travel costs are expected to increase by 5% and bus costs (fare) would be decreased by 15%.  Based on the equation above, what will the percent change in auto travel be?

Question 2 (Congestion Pricing )

Please explain (if possible with diagrams) how is the introduction of a high-occupancy lane (HOV) on a highway equivalent to congestion pricing (e.g., charging monetary toll) in order to bring efficiency (system optimality) in peak traffic flows.

 

Question 3  (UTP - Trip Distribution)

A four-zone study area has the trip productions (origins) and zonal attractiveness shown in the Table 1.   The inter-zonal and intra-zonal friction factors are as given in Table 2.

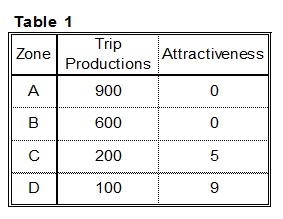
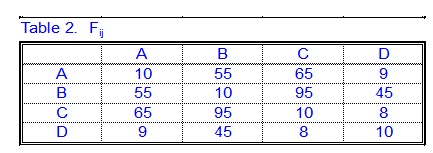
Determine all zonal interchange volumes (T_ij), using the gravity model.

 

Question 4. (Mode Choice)

A city commuter population has the following modal utility function, U_k = A_k - 0.003C - 0.04T

 

Where        A_k   constant for mode k

                 C    out-of-pocket travel cost in cents.

                 T    riding time in minutes

(a)    Apply the logit model to calculate the market shares of the two travel modes used in the city.

 

                           Mode                        A_k                     C                      T

                           Automobile               -0.20                240                  25

                           Regular city bus        -0.60                  80                  55

 

(b)   Suppose, in addition to the regular city bus, a private company starts its bus service along the same routes as the city bus with the following characteristics:

                           Mode                        A_k                     C                      T

                           Private bus                -0.60                  80                  55

 

            Determine the new market shares of auto, city bus and private bus.

 

Question 5 (10 points) (All-or-nothing / incremental assignment)

For the network shown below, 4 units of flow go from node 1 to node 3.  The average travel time functions on these links are:

t_a = 3 + X_a²

t_b = 1 + 3X_b

t_c = 2 + X_c

(a)    Assign the 4 units of flow from node 1 to node 3 using incremental ( i.e., one unit at a time) capacitated ALL-OR-NOTHING assignment technique.  Show your work step by step as well as the final link flows  (X_a , X_b , X_c ).

(b)   Are the resulting flows in system optimum or user equilibrium?  Please explain.

Question 6 (User-Equilibrium vs. System Optimum Traffic Assignment)

 

For the network shown below, the following origin-destination volumes are the only volumes to be assigned to the network:V^AC = 800               V^BC = 100      V^BD = 900

t₁  =  10 + 0.01 x₁

t₂  =    3 + 0.02 x₂

t₃  =    2 + 0.02 x₃

t₄  =    3 + 0.02 x₄

t₅  =    4 + 0.02 x₅

t₆  =  12 + 0.01 x₆

 

t₇  =    4 + 0.02 x₇

Is she correct?  Why?