Reference no: EM131032713

1. NK Model

Consider a NK model with no capital, like the model discussed in Lectures 19 and 20. In this model, households choose their money balances M_t, labor supply N_t, and savings B_t+1 in a nominal bond to solve

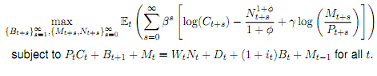
(a) As shown in Lecture 19, the household optimality or intratemporal Euler condition for labor supply N_­t is given by

1/C_t W_t/P_t = N_t^φ.

Interpret this condition in words.

(b) As shown in Lecture 19, the household optimality or intertemporal Euler condition for money balances M_t is given by

1/C_tP_t = γ1/M_t + βE_t[1/C_t+1P_t+1]

Interpret this condition in words.

(c) As shown in Lecture 19, the household optimality or intertemporal Euler condition for savings B_t+1 is given by

1/C_tP_t = β(1 + i_t+1)E_t[1/C_t+1P_t+1].

Interpret this condition in words.

(d) Using the optimality conditions for M_t and B_t+1, write a money demand equation linking the household's optimal real money balances M_t/P_t to a function of consumption and the nominal interest rate i_t+1. If the nominal interest rate i_t+1 increase, do real money balances increase or decrease? Explain the intuition for this in words.

(e) Assume that the equation Mt = ΨP_tY_t holds for some constant Ψ > 0, i.e. assume that money satisfies a constant velocity equation in this economy. Assume that prices P_t are perfectly fixed in the short run because the prices P_t are set by firms in period t - 1. If there is an unexpected increase in the money supply M_t in period t, use the constant velocity formula to determine whether output Y_t increases, decreases, or stays the same. Explain the intuition behind your result in words.

(f) Now, assume that the money supply M_t and the output level Y_t grow at constant rates, i.e. that

M_t+1 = (1 + g_M)M_t

Y_t+1 = (1 + g_Y)Y_t

for some net growth rates g_M > g_Y > 0. Use the constant velocity formula M_t = ΨP_tY_t to derive a formula for the gross inflation rate Π_t+1 = P_t+1/P_t as a function of g_M and g_Y. Then, use the log approximation log(1+ x) ≈ x to write π_t+1 = log Π_t+1 as a function of g_M and g_Y. If the money growth rate g_M increases, does inflation π_t+1 increase or decrease?

(g) Using the Fisher equation r_t+1 = i_t+1 -π_t+1 as well as your answer to part (f), write the real interest rate r_t+1 as a function of the nominal interest rate i_t+1, the money growth rate g_M, and the output growth rate g_Y. If the money growth rate g_M increases, does the real interest rate increase or decrease? If the output growth rate g_Y increases, does the real interest rate increase or decrease?

2. Optimal Monetary Policy

Consider a central bank's optimal monetary policy problem with discretion as described in Lecture 21. The central bank desires to choose the output gap x_t, the inflation rate π_t, and nominal interest rates i_t+1 in each period t in order to optimize their objective

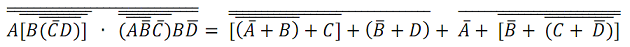
subject to cost-push shocks ε_t^s and demand shocks ε_t^d to the economy satisfying

ε_t^s = ρ^s ε^s_t-1 + v^s_t,      ε­_t^d = ρ^d ε^d_t-1 + v_t^d

as well as the IS and Phillips curves

x_t = -φ (i_t+1 - E_tπ_t+1) + E_tx_t+1 + ε_t^d

π_t = βE_tπ_t+1 + λx_t + ε_t^s.

As shown in Lecture 21, the optimal monetary policy under discretion implies that

x_t  = -λγ_sε_t^s

π_t = αγ_sε_t^s

i_t+1 = γ_π E_tπ_t+1 + (1/φ)ε_t^d

where γ_s = (1/ λ² + α(1-βρ^s)) > 0, γ_π  = (1 + (λ(1-ρ^s)/φαρ^s))  > 1, and α, λ > 0.  Assume that in period t = 0, the cost-push and demand shocks satisfy ε_t^s = ε_t^d = 0 and that the central bank is engaging in optimal monetary policy subject to discretion with x_t = π_t = 0. Then, in period 1, assume that there is a single positive cost-push innovation with υ₁^s = 1. All other shocks are zero with υ_t^s = 0 for t > 1 and υ_t^d = 0 for all t. In other words, assume that the cost-push shock ε_t^s increases unexpectedly in period 1 but that no other innovations hit the economy thereafter. Using the formula for ε_t^s above, it is possible to show under these conditions that the formula ε­_t^s = (ρ^s)^t-1 holds for all t = 1, 2, .... If 0 < ρ^s < 1, then the evolution of the cost- push shock ε_t^s as a function of t after the initial shock in period t = 0 must look something like the curve plotted in Figure 1. Note that it must be the case that ε_t^s → 0 as t → ∞.

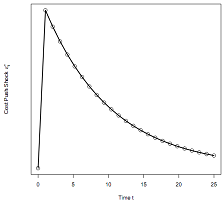
Figure: ε_t^s in Response to a Positive Cost-Push Innovation at Time t = 1

(a) Using the formula for ε_t^s given above, together with the optimal policy formula for x_t given above, draw a curve describing the evolution the output gap x_t in response to the positive cost-push innovation in period 1. In period 1, does the output gap x_t increase, decrease, or stay the same? Describe the path for the output gap x_t after the cost-push innovation in period's t > 1.

(b) Using the formula for ε_t^s given above, together with the optimal policy formula for π_t given above, draw a curve describing the evolution the inflation rate π_t in response to the positive cost-push innovation in period 1. In period 1, does the inflation rate π_t increase, decrease, or stay the same? Describe the path for the inflation rate π_t after the cost-push innovation in period's t > 1.

(c) Based on your answers in parts (a) and (b), do the output gap and inflation move in the same direction or in opposite directions in response to a cost-push innovation? Explain the intuition behind this result in words.

(d) Assume that the central banker is an extreme "inflation hawk" with   α → 0, so that the output gap and inflation coefficients -λγ_s → - (1/λ) and αλ_s → 0. Draw figures plotting the responses of the output gap and inflation to the cost-push innovation in period 1. Compare these patterns to the output gap and inflation paths from parts (a) and (b), and explain the intuition behind the differences you see.

(e) Assume that the central banker is an extreme "inflation dove" with α → ∞, so that in the limit the output gap and inflation coefficients satisfy -λγ_s → 0 and αλ_s → 1/1-βρ^s.  Draw figures plotting the responses of the output gap and inflation to the cost-push innovation in period 1. Compare these patterns to the output gap and inflation paths from parts (a) and (b), and explain the intuition behind the differences you see.

Attachment:- Assignment.rar