Determine the equations of the vertical asymptotes

Assignment Help Mathematics

Reference no: EM131547146

Determine the amplitude, range, period and phase shift of each, then sketch the graph: Include at least one full period.

1. y = 3sin( x + Π/4) +1

2. y = -cos(Π - 2x)+ 3

Determine the equations of the vertical asymptotes and all relative maximum and relative minimum points for two full periods, then sketch the graph:

3. y = -2csc(2x -Π )- 3

4. y = sec( 2x - Π/4) - 2

Determine the interval of a principal cycle and then identify the vertical asymptotes, the coordinates of the center point, and the coordinates of the halfway points. Sketch the graph.

5. y =1/2 tan ( 1/2.x + Π )

6. y = 5 + 3cot ( 3x + Π/3 )

Evaluate each to the nearest hundredth:

7. sin^-1 (0.73)

8. cot^-1 (-13)

Answer each:

9. Rewrite cot (sin^-1 2x) as an algebraic expression involving the variable x. Assume that x > 0 and the angle is in quadrant I.

10. Using the graph of y = sin x , find all the exact values of x on the interval [- 7Π/2 , 5Π/3 ] that satisfy the ordered pair (x, √3/2).

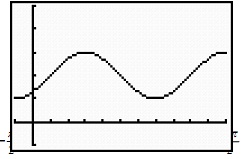
11. Write the cosine function to fit the given graph:

Reference no: EM131547146

Questions Cloud

Relationship between discrimination and prejudice : Explain the relationship between discrimination and prejudice. Provide an example that you have experienced.

Race and ethnic relations that brazil might face : Do you think there are any foreseeable challenges surrounding race and ethnic relations that Brazil might face?

Calculate rate of return over its useful life : Assuming an effective federal tax rate of 25%, and state tax rate of 5%, calculate the rate of return over its useful life.

Assume annual compounding on the zero coupon bond : what will your company's repayment be if you issue the coupon bonds? What if you issue the zeroes?

Determine the equations of the vertical asymptotes : Determine the amplitude, range, period and phase shift of each, then sketch the graph: Include at least one full period - Determine the equations of asymptotes

What is the ending accounts payable balance : What is the ending accounts payable balance? What is the ending equipment balance net of accumulated depreciation? What is the cost of goods manufactured?

Calculate the payout ratio : What percentage of Apple's authorized common stock was issued at September 27, 2014?

About the usual overall of maximizing shareholder value : What about ethics, are they about the usual overall of maximizing shareholder value or practicing the values of the majority shareholders.

About the NPV and IRR Analysis : After discovering a new gold vein in the Colorado mountains, CTC Mining Corporation must decide whether to go ahead and develop the deposit.

User Account

All Pages