Reference no: EM131757742

Question: Mr. Schellburger, the CEO of Schellburger Oil, is facing a dilemma-he is not sure whether or not he should drill an oil well. His decision situation is captured by the following decision tree (all prospects are expressed in millions of dollars);

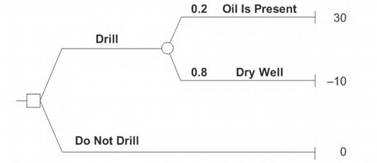
Fortunately, Mr. Schellburger has access to a detector which can give him some further information on whether or not there the site contains oil. The detector is symmetric and has accuracy 0.6.

Mr. Schellburger's engineering team tells him that he can use the detector as it is, or they can improve it for him in one of three ways before he uses it:

I. They can improve { "Oil Is Present" | Oil Is Present, & } to be equal to 1, at a cost of $3 M.

II. They can improve { "Dry Well" | Dry Well, & } to be equal to 1, at a cost of $3 M.

III. Or, they can improve both of those probabilities to be equal to 1, at a cost of $6 M.

Mr. Schellburger follows the delta property and his risk tolerance is $200 M. What should he do?

a. Use the detector in its present state, without any improvements.

b. Improve { "Oil Is Present" | Oil Is Present, & } before using the detector.

c. Improve { "Dry Well" | Dry Well, & } before using the detector.

d. Improve both probabilities before using the detector.