Create the virtual domain of the m-shaped tensile test

Reference no: EM132478706

Problem 1: The meshed model of a bath is shown in Figure CW2-2. The bath is made of steel of Young's Modulus, E = 210 GPa. The mesh is based on 2D quadrilateral element. We are interested in developing the element formulation for our bespoke four-node quadrilateral element whose isoparametric natural coordinates are given in Figure CW2-2.

For the quadrilateral element:

i. Show derivations of the relevant element shape functions, Ni where i = 1, 2, 3, 4, needed to describe the element formulation for the quadrilateral element.

ii. The plot of the four shape functions

iii. Derive the resulting strain-displacement matrix, B of this element type.

iv. Using the B-matrix above and material properties, derive the applicable stiffness matrix, K that will be used within the finite element solver for this new element type.

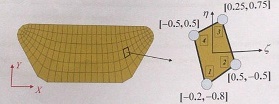
Figure CW2-2: A bath discretized with quadrilateral element. Insert shows the isoparametric natural coordinates of the bespoke quadrilateral element.

Problem 2:
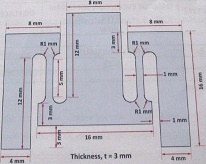
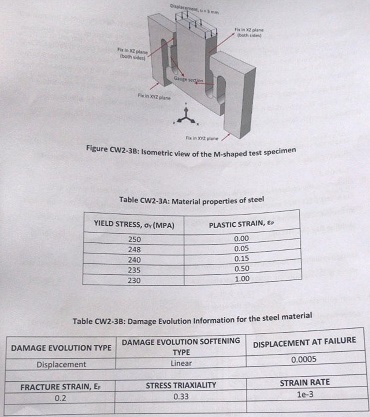
An m-shaped specimen, shown in figure CW2-3A, is used to determine the dynamic tensile behaviour of a steel specimen within a split Hopkinson Pressure Bar apparatus. The dimensions of the test specimen are given in Figure CVV2-3A and the specimen is made from steel with properties given In Table CW2.3A.

The upper end of the specimen is imposed with a compressive displacement. U = 3 mm while both bases are fixed securely in all X, Y and Z-axes. The side faces are fixed in XZ-plane allowing for movement only in the Y-axis. The steel plate is to be modelled using an elastoplastic material model with isotropic softening given in Table CW2-3A and damage evolution law with properties given in Table CW2-38.

i. Create the virtual domain of the M-shaped tensile test specimen in an appropriate software of your choice. Create a nodal set for the gauge sections of the test specimen.

ii. Undertake the compressive test simulation for the specimen using hexahedral elements with ELEMENT DELETION switched to ON. Show contour plots from your simulation for the Von Mises stress (MISES), equivalent plastic strain (PEEQ), displacement, (U MAGNITUDE) and actively yielding flag (AC YIELD).

Using simulation outputs, determine the plot of volume averaged y-stress (S22) versus y-axis strain stress (E22) for only the gauge section elements.

From your simulations, calculate the error between numerical and analytical yield stresses determined from your study? Discuss three sources of the errors from your study.

Reference no: EM132478706

Questions Cloud

Difference in tools and personnel into a network environment : Discuss some of the differences in tools, techniques, skills and personnel that are needed between an intrusion into a network environment and a response to a.

What is the interest expense to be recognized for year two : The bond is issued for 90 percent of face value ($72,000) to yield an annual rate of 8 percent. What is the interest expense to be recognized for Year Two

How is monopolistic competition similar : How is monopolistic competition similar to each of the following market structures?

How cost of security controls implementation was justified : In 80 words or more give an example of a cost analysis conducted within your organization, and how the cost of security controls implementation was justified?

Create the virtual domain of the m-shaped tensile test : Create the virtual domain of the M-shaped tensile test specimen in an appropriate software of your choice. Create a nodal set for the gauge sections of the test

How much money could be saved each year in interest expense : If all freed up dollars would be used to pay down debt that has an interest rate of 8%, how much money could be saved each year in interest expense?

Discussion about the risk tolerance : In 80 words or more give an example of risk tolerance documents and how it has shaped the IT security model within your organization?

Draw a correctly labeled graph for a monopoly : a) Draw a correctly labeled graph for a monopoly and show each of the following.

Determine the dividends per share for preferred stock : Sabas Company has 20,000 shares of $100 par, Determine the dividends per share for preferred and common stock for the third year.

User Account

All Pages