Reference no: EM131177
Problem 1
Solve the following problem graphically (Please be neat) (Suggestion, use some graphing software such as GraphCalc® to create the feasible solution space. Then print that graph for your analysis):
Maximize Z = 8x1 + 5x2
Subject to:
-4x1 + x2 ≤ 1
3x1 + 4x2 ≤ 25
5x1 -2x2 ≤ 12
-1.5x1 + 3x2 ≤ 8
and
x1,x2 ≥ 0
Problem 2
Work through the simplex method (in algebraic form) step by step to solve the following problem. Show all work and provide the solution.
Maximize Z = x1 + 3x2 + 2x3,
subject to
5x1 + 2x2 + 3x3 ≤ 15
x1 + 4x2 + 2x3 ≤ 12
2x1 + x3 ≤ 8
and
x1 ≥ 0, x2 ≥ 0, x3 ≥ 0.
Problem 3
My diet requires that all food I eat come from one of the four "Basic food groups" (chocolate cake, ice cream, soda, and cheesecake). At present, the following four foods are available for consumption: brownies, chocolate ice cream, cola, and pineapple cheesecake. Each brownie costs 50¢, each scoop of chocolate ice cream cost 20¢, each bottle of cola cost 30¢, and each piece of pineapple cheesecake cost 80¢. Each day, I must ingest at least 500 calories, 6 oz of chocolate, 10 oz of sugar, and 8 oz of fat. The nutritional content per unit of each food is shown in the following table. Formulate a linear programming model that can be used to satisfy my daily nutritional requirements at minimum cost.

1. Formulate a linear programming model for this problem by:
A. Listing and labeling all of the decision variables.
B. Creating an objective function for the model.
C. List all
2. Solve the model using Excel® solver. Give the value for each decision variable and the objective function.
Problem 4.
The Weigelt Corporation has three branch plants with excess production capacity. Fortunately, the corporation has a new product ready to begin production, and all three plants have this capability, so some of the excess capacity can be used in this way. This product can be made in three sizes--large, medium, and small--that yield a net unit profit of $420, $360, and $300, respectively. Plants 1, 2, and 3 have the excess capacity to produce 750, 900, and 450 units per day of this product, respectively, regardless of the size or combination of sizes involved.
The amount of available in-process storage space also imposes a limitation on the production rates of the new product. Plants 1, 2, and 3 have 13,000, 12,000, and 5,000 square feet, respectively, of in-process storage space available for a day's production of this product. Each unit of the large, medium, and small sizes produced per day requires 20, 15, and 12 square feet, respectively.
Sales forecasts indicate that if available, 900, 1,200, and 750 units of the large, medium, and small sizes, respectively, would be sold per day.
At each plant, some employees will need to be laid off unless most of the plant's excess production capacity can be used to produce the new product. To avoid layoffs if possible, management has decided that the plants should use the same percentage of their excess capacity to produce the new product.
Management wishes to know how much of each of the sizes should be produced by each of the plants to maximize profit.
1. Formulate a linear programming model for this problem by:
D. Listing and labeling all of the decision variables.
E. Creating an objective function for the model.
F. List all
2. Solve the model using Excel® solver. Give the value for each decision variable and the objective function.
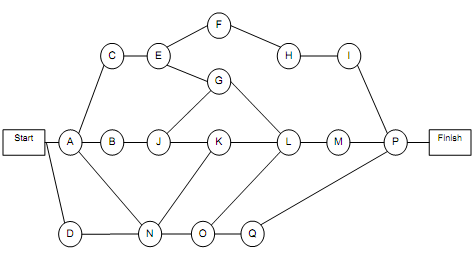
Problem 5. For the project schedule, answer the following questions.
1. What is the total time required to complete the project if no delays occur?
2. When do the individual activities need to start and finish (at the latest) to meet this project completion time?
3. When can the individual activities start and finish (at the earliest) if no delays occur?
4. Which are the critical bottleneck activities where any delays must be avoided to prevent delaying project completion?
5. For the other activities, how much delay can be tolerated without delaying project completion?

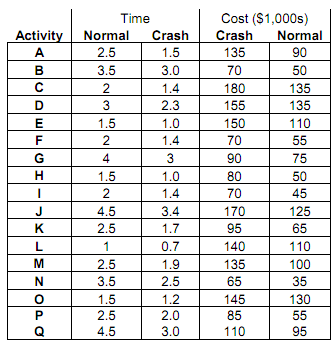
6. Suppose the following data is given pertaining to the cost associated with crashing activities. What activities should be crashed and how much will it cost if the deadline is changed to:
a. 19 weeks
b. 18.5 weeks
c. 18 weeks
d. 17.5 weeks
e. 17 weeks
f. 16.5 weeks
g. 16 weeks
h. 15.5 weeks
i. 15 weeks
7. Plot the cost of time reduction against total time reduction and draw any conclusions. What happens to the cost as the amount of time reduction is increases?