Calculate the rotation at point a and b using virtual work

Reference no: EM1377050

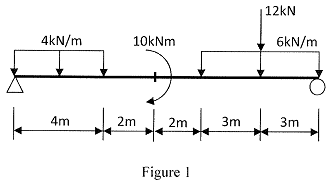
1(a) Draw the FBD and the shear and bending moment diagrams for the beam shown in Figure I. Label all minimums, maximums and zero crossing points as well as the slopes as constant, linear or parabolic.

509_shear and bending moment diagrams.png

2. A simply supported beam is shown in Figure 2(a), and the cross-section is given in Figure 2(b).

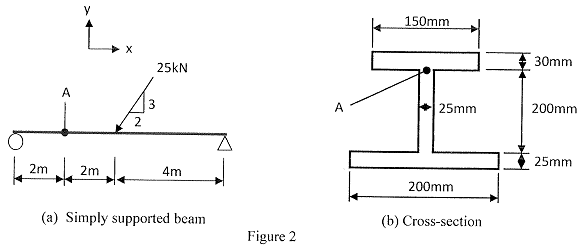
1358_shear and bending moment diagrams1.png

(a) Draw the F130 for the structure and solve for the reactions. Cut the structure at point A (as seen in Figure 2(a)) and draw the FBI) and solve for the forces at point A.

(b) Use the parallel axis theorem to solve for the second moment of area, I, of the cross-section in
Figure 2(b).

(c) Solve for the normal stress and shear stress in the x and y directions and the principal stresses at point A (as illustrated in Figures 2(a) and 2(b)).

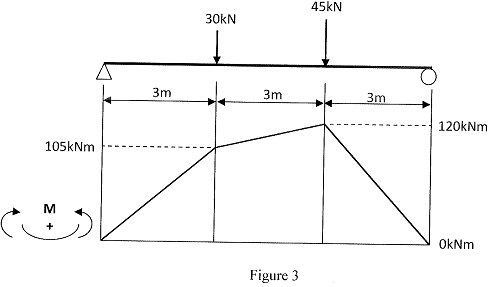
3. Use the moment area method to determine where along the beam, shown in Figure 3, the maximum deflection of the beam occurs using the moment diagram provided. Assume El = 1 x 10¹³ Nmmz. DO NOT solve for the maximum deflection.

317_shear and bending moment diagrams2.png

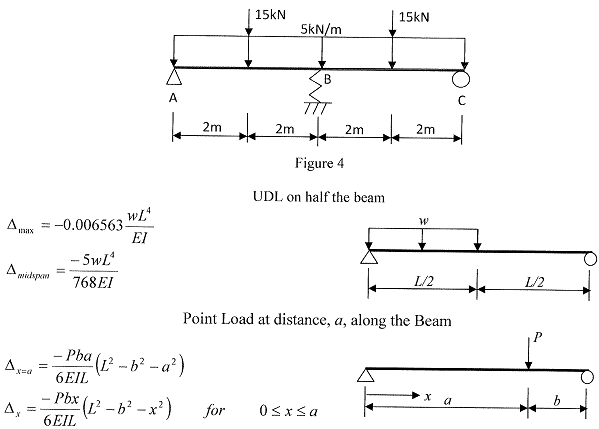
4. The statically indeterminate beam in Figure 4 is supported by a pin at A, a spring with stiffness of 10000 N/mm at B and a roller at C. Use the force method to solve for the reactions at A, B and C. Assume El = 2 x 10¹³Nmm². The deflection equations for some common beams are also given below.

1874_shear and bending moment diagrams3.png

5. The column shown in Figure 5(a) is pinned at the bottom and is restrained against horizontal movement at the top. Based on the cross-section in Figure 5(b), a yield strength of 300 MPa, and a modulus of elasticity of 200 GPa, determine whether a load of P = 250kN with an eccentricity of 150 mm to the weak axis can be applied to the column safely.

1222_shear and bending moment diagrams4.png

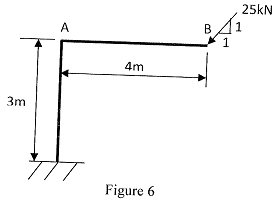
6. For the frame shown in Figure 6, calculate the rotation at point A and B using virtual work. In your expert opinion, do these rotations seem reasonable? Assume El = 3 x 10¹³nmm².

15_shear and bending moment diagrams5.png

Reference no: EM1377050

Questions Cloud

Calculate labor productivity per labor hour : David Upton is leader of Upton Manufacturing, a manufacture of Go-Kart tires. Upton makes 1,000 tires per day with the given resources:

Discuss major issues affect moral, productivity and safety : Scheduling people to work late, or "graveyard", shift is a problem in almost every twenty-four hour company.

Determine the multifactor productivity ratio : Med-Tech labs is a facility that gives medical tests and evaluations for patients ranging from examining blood samples to performing MRI's.

Annual performance appraisals : Describe how a complete performance management system differs from the use of yearly performance appraisals and explain the advantages of managing turnover in organizations

Calculate the rotation at point a and b using virtual work : Draw the FBD and the shear and bending moment diagrams. Label all minimums, maximums and zero crossing points as well as the slopes as constant, linear or parabolic.

Determine the proportion of time : As a class assignment you have been asked to assignment the proportion of time a professor spends on various activities. You have decided to use work sampling method

Interest based negotiation and large group methods : Discuss the similarities and differences between interest based negotiation and large group methods like future search.

Question about pollution : Suppose you live in a city with a strong petrochemical industry. Each time you pass through a certain part of town, you smell a noxious odor.

Discuss the process strategy : Determine where is the headquarter of McDonald's and are there any benefits locating there? If you could choose, where would you select and why?

User Account

All Pages