Reference no: EM13704693
Problem:
Question 1: Using the method of characteristic calculate the flow in a straight wall channel with 9 degrees inclineand inlet M=1.439. Use four points across the channel as shown. Show your results in a tabular form similar to Table 11.1Noncircular Torsion 1.
A 3 in. x 6 in. wood member AB is rigidly supported at B. A 15 in. bracket is attached at the other end and the load W is applied. We define the stiffness here as the end force to produce a unit downward deflection of the bracket at the load. Take G = 500 ksi (G for wood is not usually tabulated). Neglect the deformation of the bracket. Do not include the bending of the wood member, only its twisting.

Determine the stiffness and the maximum allowable end deflection if the shear stress in the board is not to exceed 900 psi.
Thin-Walled Torsion Theory
Question 2: 'Modified from another source.] A hollow extruded shaft has the cross-section shown below. The material is aluminum with Young's Modulus 70.0 GPa and Poisson Ratio 0.33.
a) If we wish to make sure the maximum shear stress does not exceed 240 MPa, determine the largest torque that can be applied to the cross-section. Neglect stress concentrations.
b) Using the value of torque from part a, determine the 'rate of twist' de/dz. Use units of degrees per inch. As part of your work, please provide a numerical value for the torsion constant, J.
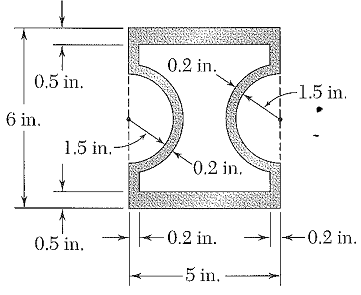
Question 3: The two-cell, three-stringer beam with the cross-section shown in the figure subject to an applied torque, T, of 200 kN-m. Assume the "nose" is semicircular. The material is Al 2024-13. Note: the stringers (longitudinal stiffeners) do not appreciably influence the torsion behavior and can be neglected in this problem.
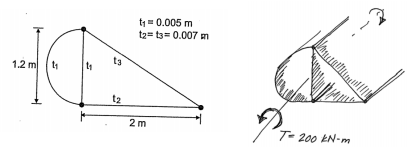
a) Find the shear flow acting in each web/skin segment in the structure. As part of your final answer, in a sketch, clearly illustrate the resultant shear flow acting in each segment of the wall indicating its numerical value and direction.
b) Determine the "rate of twist" (de/dz) for the structure.