Transformation of the independent variable

Shifting and folding (reflecting about the vertical axis)
Given the sequence x(n), here n is the independent variable, we have the 2 transformation operations as stated below:

• Shifting in time by k units, where k is an integer, is represented by x(n-k). The sequence x(n-k) represents sequence x(n) shifted by k samples, to right if the k is positive, or to the left if the k is negative in nature. Parenthesize n and then replace it by (n-k) for the k units of delay, or by (n+k) for the k units of time advancement.
• Folding (a.k.a. time reversal or reflecting about the vertical axis) can be denoted by x(-n). The signal x(-n) corresponds to reflecting x(n) about the time origin n = 0. Now reverse the sign of n (replace n with -n).

As in the case of continuous-time signals the operations of shifting and folding are not commutative. In other words, the result of first shifting and then folding is not the same as that of first folding and then shifting.

The 3rd operation is scaling, which is discussed later in this unit.

Example Delta function- Given the delta function δ(n), first it reflects and then shifts by 2 units. The other possibility is 1st to shift by 2 units and then reflect.

The result of "reflect then shift" is shown below left. The word Reflect means to change the sign of n; then shifting can be done by replacing (n) by (n-2). The result is: (1) δ(n) → δ(-n) and (2) δ(-n) = δ(-(n))→ δ(-(n-2)) = δ(-n+2).

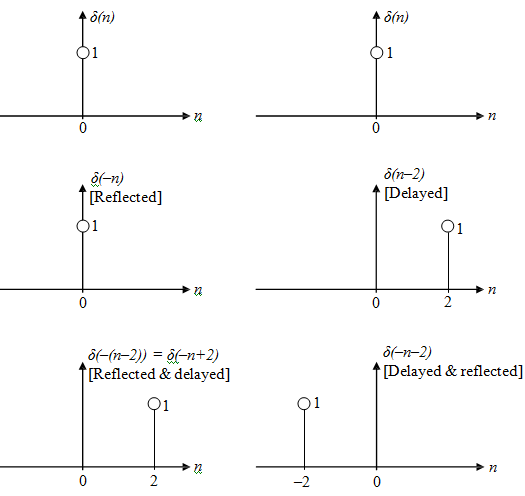
By continuing the example, the second possibility, the result of "shift then reflect" can be shown above right. Shift means replacing (n) by (n-2); then reflects by changing the sign of n. The result is δ(- n-2). 

The result is: (1) δ(n) = δ((n))→ δ((n-2)) and (2) δ((n-2)) = δ(n-2) → δ(-n-2).

Note that the 2 end results are not the same. This serves to show that the 2 operations of shifting and reflecting are not commutative in nature:

Fold(Shift(δ(n))) ≠ Shift(Fold(δ(n)))

Email based Transformation of the independent variable assignment help - Transformation of the independent variable homework help at Expertsmind

Are you finding answers for Transformation of the independent variable based questions? Ask Transformation of the independent variable questions and get answers from qualified and experienced  Digital signal processing tutors anytime from anywhere 24x7. We at www.expertsmind.com offer Transformation of the independent variable assignment help -Transformation of the independent variable homework help and  Digital signal processing  problem's solution with step by step procedure.

Why Expertsmind for Digital signal processing assignment help service

1.     higher degree holder and experienced tutors

2.     Punctuality and responsibility of work

3.     Quality solution with 100% plagiarism free answers

4.     On Time Delivery

5.     Privacy of information and details

6.     Excellence in solving Digital signal processing queries in excels and word format.

7.     Best tutoring assistance 24x7 hours