Spectrum of a down-sampled signal provided the signal x(n) whose spectrum is X(ω) or X(ejω) we need to search the spectrum of y(n), the down-sampled version of x(n), shown by y(n) ↔ Y(ω).
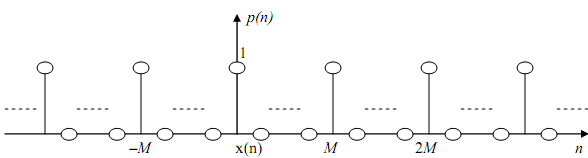
Suppose the periodic train of impulses, p(n), with period M
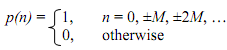
The discrete Fourier series shows of p(n) is
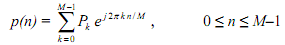
The Fourier coefficients are provided by
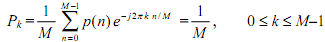
Thus the DFS for p(n) is

Describe the signal x'(n)

The sequence x¢(n) consists of values of x(n) whenever n = 0, ±M, ±2M, ..., and zeros in between that points.
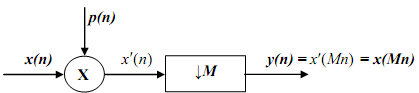
Describe the down-sampled version y(n)
y(n) = x'(Mn) = x(Mn) p(Mn) = x(Mn)
The signal y(n) has of values of x(Mn) at n = 0, ±1, ±2, ..., but no zeros in between.
With y(n) = x'(Mn) = x(Mn) our objective is to search the spectrum Y(ω).Take in mind that X(ω) periodic in ω since x(n) is a discrete-time sequence; and the similar is true of Y(ω). Now the z-transform of y(n) is
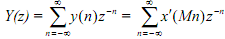
Set Mn = k: then n = k/M and the summation limits n = {- ∞ to ∞} become k = {- ∞ to ∞}. Thus
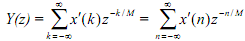
Here x'(n) = 0 except when n is a multiple of M. Substituting x(n) p(n) for x'(n) in the above equation,
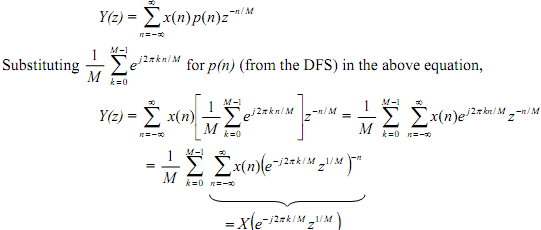
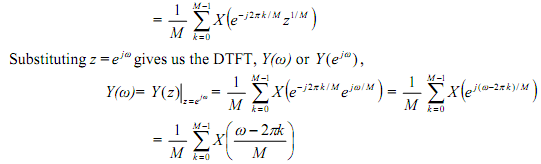
Email based Spectrum of a down-sampled signal assignment help - Spectrum of a down-sampled signal homework help at Expertsmind
Are you finding answers for Spectrum of a down-sampled signal based questions? Ask Spectrum of a down-sampled signal questions and get answers from qualified and experienced Digital signal processing tutors anytime from anywhere 24x7. We at www.expertsmind.com offer Spectrum of a down-sampled signal assignment help -Spectrum of a down-sampled signal homework help and Digital signal processing problem's solution with step by step procedure.
Why Expertsmind for Digital signal processing assignment help service
1. higher degree holder and experienced tutors
2. Punctuality and responsibility of work
3. Quality solution with 100% plagiarism free answers
4. On Time Delivery
5. Privacy of information and details
6. Excellence in solving Digital signal processing queries in excels and word format.
7. Best tutoring assistance 24x7 hours