Proper rational function Taking a0 = 1, we have
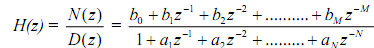
This is called as proper rational function if aN ≠ 0 and M < N. This amounts to saying that the number of finite zeros is less than the number of finite poles. This condition is related to partial fraction expansion and has nothing to do with causality.
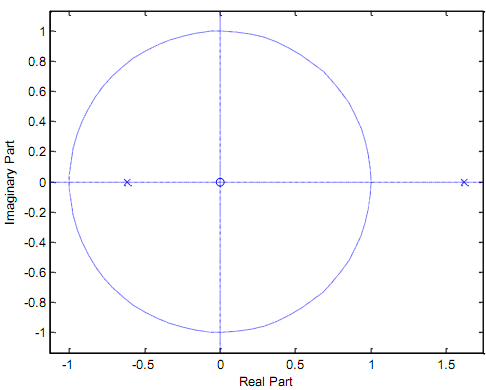
Example Give pole-zero plot for H(z) = 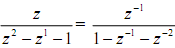
Solution The denominator has roots or poles at
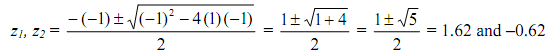
There is a zero at z = 0. Further, as the denominator degree is greater than numerator degree by 1 it is clear that H(∞) = 0, such that there is an additional zero at z = ∞.
In MATLAB the transfer function can be specified as a ratio of polynomials in z-1
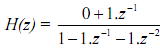
Numerator coefficients, {bi, i = 0 to M} and denominator coefficients {ai, i = 0 to N} are specified as the 2 vectors b = [0, 1] and a = [1, -1, -1].
%Pole-zero plot
b = [0, 1]; a = [1, -1, -1]; zplane (b, a)
Email based Proper rational function assignment help - Proper rational function homework help at Expertsmind
Are you finding answers for Proper rational function based questions? Ask Proper rational function questions and get answers from qualified and experienced Digital signal processing tutors anytime from anywhere 24x7. We at www.expertsmind.com offer Proper rational function assignment help -Proper rational function homework help and Digital signal processing problem's solution with step by step procedure.
Why Expertsmind for Digital signal processing assignment help service
1. higher degree holder and experienced tutors
2. Punctuality and responsibility of work
3. Quality solution with 100% plagiarism free answers
4. On Time Delivery
5. Privacy of information and details
6. Excellence in solving Digital signal processing queries in excels and word format.
7. Best tutoring assistance 24x7 hours