Problems related to series of binomial coefficients:

 Problems having binomial coefficients with alternate sign:

Illustration: Calculate C₀ - C₁ + C₂ - C₃ +...+ (-1)ⁿC_n.

Solution: Here alternately +ve and - ve sign occur

               That may be obtained by putting (-1) instead of 1 in place of x in

               (1 + x)ⁿ = C₀ + C₁x +...+ ⁿC_nxⁿ, we get C₀ - C₁ +...+ (-1)ⁿC_n = 0

               Now to obtain the sum C₀ + C₂ + C₄ + ...

               we add (1 + 1)ⁿ and (1 - 1)ⁿ.

                                    Similarly, the cube roots of unity can be used to calculate

                                    C₀ + C₃ + C₆ + ... OR C₁ + C₄ +... OR C₂ + C₅ +...

                                    put x = 1, x = w, x = w² in

                                    (1 + x)ⁿ = C₀ + C₁x +...+ C_nxⁿ and include to obtain C₀ + C₃ + C₆ +...

                                    the other two can be calculated by suitably multiplying (1 + w)ⁿ and

                                    (1 + w²)ⁿ by w and w² respectively.

•  Problems  belong  to series of  Binomial coefficients in which each and every term is a product of  an integer and a binomial coefficient i.e. in the  form  k ⁿC_r.

Illustration: If (1+x)ⁿ = 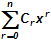  then  show  that C₁ + 2C₂ + 3C₃+. . .+ nC_n= n2^n-1.

Solution:  Method (i): By addition

                                    r^th term of the provided series, t_r = r ⁿC_r => t_r = n ^n-1C_r-1

                                    Sum of  the series =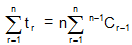

            = = n 2^n-1 .

              Method (ii) By calculus

We  have ( 1+ x )ⁿ = C₀ + C₁x + C₂ x² +  . . . + C_nxⁿ         . . .(1)

Differentiating  (1)  with  related  to x 

n(1 +x )^n-1   = C₁  +2C₂x  + 3C₃ x² +  . . . + n C_nx^n-1           . . . (2)

Putting  x = 1 in (2),  n 2^n-1 = C₁ + 2C₂ + . . .  + n ⁿC_n 

• Problems related  to  series  of  binomial  coefficients in  which  each and every  term is a binomial  coefficient  divided by an integer i.e. in the   form of  .

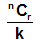Email based Problems related to series of binomial coefficients Assignment Help - Homework Help

We at www.expertsmind.com offer email based Problems related to series of binomial coefficients assignment help - homework help and projects assistance from k-12 school level to university and college level and engineering and management studies. We provide finest service of Mathematics assignment help and Mathematic homework help. Our experts are helping students in their studies and they offer instantaneous tutoring assistance giving their best practiced knowledge and spreading their world class education services through e-Learning program.

Expertsmind's best education services

• Quality assignment-homework help assistance 24x7 hrs
• Best qualified tutor's network
• Time on delivery
• Quality assurance before delivery
• 100% originality and fresh work