Orthogonal intersection of two circles:

The 2 circles are said to intersect orthogonally if angle between the tangents at their point of intersection is equal to 90⁰.

The condition for 2 circles S₁=O and S₂=O to cut each other orthogonally is given by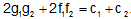

Note:  It the 2 circles are intersecting orthogonally the tangent to one circle at the point of intersection passes through the centre of the other circle.

Case V:   It the distance between centres is less than the difference of their radii that is |O₁ O₂| < |r₁-r₂|, in this case one circle will lie inside the other circle. Therefore there will be no common tangent.

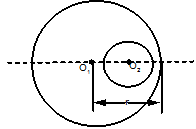
Illustration: If the circles x² + y² + 2ax + 2by = 0 and x² + y² + 2bx + 2cy = 0 touch each other, then show that b²=ac.

Solution : Both the circles are touching each other. Thus distance between the centres should be equal to sum of the radii. Centre are (-a, -b) and (-b, -c) and their radii are  respectively

                                                squaring both the sides

                                                => b⁴ - 2acb² + a²c² = 0       => (b² - ac)² = 0 => b² = ac

Solution: Clearly both the circles are passing through origin. Thus they will be ouching each other at (0, 0). Therefore at (0, 0) they will be having a common tangent.

                                                => 0.x + 0.y + a(x + 0) + b(y + 0) = 0 => ax + by = 0          ...(1)

                                                =>  0.x + 0.y + b(x + 0) + c(y + 0) = 0 => bx + cy = 0         ...(2)

                                                (1) and (2) identical

                                                a/b = b/c => b² = ac

Email based Orthogonal intersection of two circles Assignment Help - Homework Help

We at www.expertsmind.com offer email based Orthogonal intersection of two circles assignment help - homework help and projects assistance from k-12 school level to university and college level and engineering and management studies. We provide finest service of Mathematics assignment help and Mathematic homework help. Our experts are helping students in their studies and they offer instantaneous tutoring assistance giving their best practiced knowledge and spreading their world class education services through e-Learning program.

Expertsmind's best education services

• Quality assignment-homework help assistance 24x7 hrs
• Best qualified tutor's network
• Time on delivery
• Quality assurance before delivery
• 100% originality and fresh work