Inverse function:

If f: X→Y be a function given by y  = f(x) such that f is both one - one and onto, then there exists a general  function g: Y→X such that for each y∈Y, g(y)  =  x if  and only if y  =  f(x). The function g so defined is known as the inverse of f and indicated by f^-1.

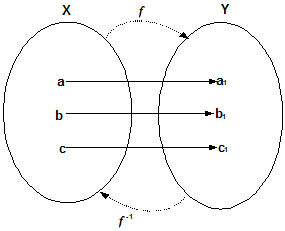
                         f(a)  =  a₁=> f ^{- 1}(a₁)  =  a

SOME BASIC POINTS:

• The situation for existence of inverse of a function is that the function have to be one - one and onto.
• Whenever an inverse function is described, the range of the real function becomes the domain of the inverse function and domain of the real function becomes the range of the inverse function.
• Note that fof ^-1(x) = f ^-1of(x) = x usually and roots of the equation f(x) = f ^-1(x) could always lie on the line  y  =  x.
• f and f ^-1 are symmetric  with respect to the line y  =  x.

Problem: The function ¦: [1, ∞) -> [1, ∞) is shown by ƒ(x)=2^{x(x - 1)}, Calculate ƒ^{- 1}(x).

Key concept : First verify the function for one - one and onto. And if function is one - one and onto then calculate inverse using the identity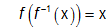 

Solution:             shown, ƒ(x)  =  2^{x(x - 1) =>} log ƒ(x)  =  x(x  -  1) log_e2

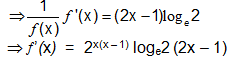
                              Therefore ƒ(x) is an increasing function in [1, ∞), thus, ƒ(x) is a one - on function.

                              Also range of f(x) is [1,∞) which is same to co - domain.

                              Therefore the function is also onto.

TO CALCULATE ƒ ^{- 1}(x):

               Suppose f ^{- 1} be the inverse function of f, then by principle of identity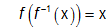 

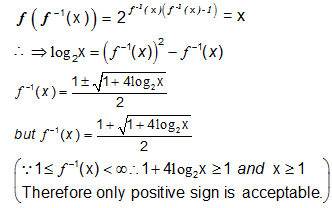
Email based Inverse function Assignment Help -Inverse function Homework Help

We at www.expertsmind.com offer email based Inverse function assignment help - homework help and projects assistance from k-12 school level to university and college level and engineering and management studies. We provide finest service of Mathematics assignment help and Mathematic homework help. Our experts are helping students in their studies and they offer instantaneous tutoring assistance giving their best practiced knowledge and spreading their world class education services through e-Learning program.

Expertsmind's best education services

• Quality assignment-homework help assistance 24x7 hrs
• Best qualified tutor's network
• Time on delivery
• Quality assurance before delivery
• 100% originality and fresh work