Greatest binomial coefficient:

In the binomial expansion of (1 + x)ⁿ , when n is even, the largest binomial coefficient is provided by ⁿC_n/2.

Same as if n be odd, the largest binomial coefficient may be 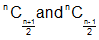 both being same.

If t_r and t_{r + 1} be the r^th and (r + 1)^th term in the expansion of (1 + x)ⁿ, then

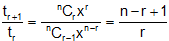x.

Suppose numerically, t_{r + 1} be the largest term in the given expansion. Then t_{r + 1} ³ t_r

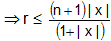                                  ......(2)

Now shifting values of n and x in (2), we obtain r £ m + f or r £ m

Where m is a positive integer, f is a fraction so that 0 £ f < 1.

Now if f = 0 then t_{m + 1} and t_m both the parts will be numerically same and bigger while if f ¹ 0, then t_{m +1} is the greatest term of the binomial expansion.

i.e. to search the largest term (numerically) in the expansion of (1 + x)ⁿ.

(i) Search m = .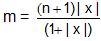

(ii) If m is integer, then t_m and t_{m + 1} are same and are biggest term.

(iii) If m is not integer, then t_{[m] + 1} is the biggest term (where [.] shows the greatest integer function).

Illustration: Calculate the value of the greatest term in the expansion of .

Solution:                   Since 

                                    t_r+1 ≥ t_r    if only 21 - r ≥r√3

                                    if only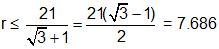

                                    Therefore t₁ < t₂ < t₃ < t₄ < t₅ < t₆ < t₇ < t₈ > t₉ > t₁₀

                                    Therefore t₈ is the greatest term and its value is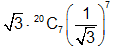 

                                                = 

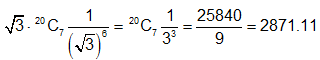
Email based Greatest binomial coefficient Assignment Help -Greatest binomial coefficient Homework Help

We at www.expertsmind.com offer email based Greatest binomial coefficient assignment help - homework help and projects assistance from k-12 school level to university and college level and engineering and management studies. We provide finest service of Mathematics assignment help and Mathematic homework help. Our experts are helping students in their studies and they offer instantaneous tutoring assistance giving their best practiced knowledge and spreading their world class education services through e-Learning program.

Expertsmind's best education services

• Quality assignment-homework help assistance 24x7 hrs
• Best qualified tutor's network
• Time on delivery
• Quality assurance before delivery
• 100% originality and fresh work