Ellipse:
An ellipse is locus of a point which moves in the plane such that the ratio of the distances from a fixed point (called as focus) and from the fixed straight line (called as directrix) is always constant and less than 1. And this constant ratio is called as eccentricity of ellipse.
Standard equation of the ellipse
|
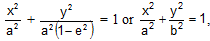
here b2 = a2(1 - e2).
The eccentricity of ellipse 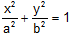 can be given by the relation b2 = a2(1 - e2), i.e., e2 = 1 - b2/a2
|
|
An ellipse has 2 foci and 2 directrices.
Latus Rectum: Latus rectum is line which passes through the focus of ellipse and perpendicular to major axis. End points of latus rectum are given by(ae, b2/a) and (ae,-b2/a).The length of semi latus rectum can be given by b2 / a.
|
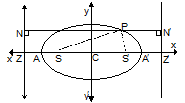
Focal Distance of a Point: To find distance of any point on the ellipse from the focus, we use definition of ellipse.
Let P(x, y) be a point on ellipse. Then
S¢P = ePN' = e(a/e -x) = a - ex
SP = ePN = e ( a/e + x) = a + ex
S'P + SP = 2a
Þ The sum of the focal distances of any point on ellipse is equal to its major axis. Also SS'<SP+S'P=2a.
|
|
|
|
Other Forms of the Ellipse:
(i) If in equation 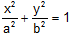, a2< b2 , then major and minor axis of ellipse lie along the y and x -axis and are of lengths 2b and 2a respectively. The foci become (0, ± be) , and directrices become y = ± b/e
here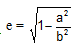 
. The length of semi-latus rectum becomes a2/b.
(ii) If centre of the ellipse be taken (h, k) and axes parallel to x and y-axes, then the equation of ellipse is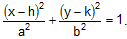 
.
Example: Find out the equation of ellipse referred to its centre whose foci are points (4, 0) and (-4, 0) and whose eccentricity is 1/3.
Solution: Let equation to the ellipse be 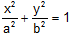......(1)
Distance between foci = 2ae = 4 + 4 = 8 ......(2)
Putting value of e = 1/3 in (2), a = 4/e = 12
Again b2 = a2(1 -e2) = 144 (1-1/9) = 128
Put in (1) we have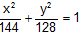 
=> 8x2 + 9y2 = 1152
Email based Ellipse Assignment Help -Ellipse Homework Help
We at www.expertsmind.com offer email based Ellipse assignment help - homework help and projects assistance from k-12 school level to university and college level and engineering and management studies. We provide finest service of Mathematics assignment help and Mathematic homework help. Our experts are helping students in their studies and they offer instantaneous tutoring assistance giving their best practiced knowledge and spreading their world class education services through e-Learning program.
Expertsmind's best education services
- Quality assignment-homework help assistance 24x7 hrs
- Best qualified tutor's network
- Time on delivery
- Quality assurance before delivery
- 100% originality and fresh work