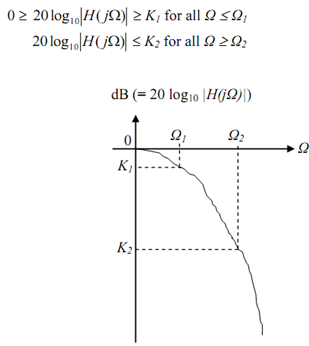
Determining the order and transfer function from the specifications A typical magnitude response specification is sketched below. The magnitudes at the critical frequencies Ω₁ and Ω₂ are A and B, respectively. Typically Ω1 is in the pass band or is the edge of the pass band and Ω₂ is in the stop band or is the edge of the stop band. For illustrative purposes we have arbitrarily

 

taken A = 0.707 (thus Ω₁ is the cut-off frequency, but this need not be the case) and B = 0.25.

The log-magnitude specification is diagrammed below. Note that (20 log A) = K₁ dB and (20 log B) = K₂ dB. Thus the analog filter specifications are

 
 With the magnitude |H(jΩ)| given by the Butterworth function,
 
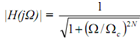
and using the equality condition at the critical frequencies in the above specifications the order, N, of the filter is given by

 

The result is rounded to the next larger integer. For example, if N = 3.2 by the above calculation then it is rounded up to 4, and the order of the required filter is N = 4. In such a case the resulting filter would exceed the specification at both Ω₁ and Ω₂. The cut-off frequency Ω_c is determined from one of the two equations below.
 

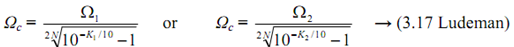
The equation on the left will result in the specification being met exactly at Ω₁ while the specification is exceeded at Ω₂. The equation on the right results in the specification being met exactly at Ω₂ and exceeded at Ω₁.

  Note that the design equation for N may be written in the alternative form
 
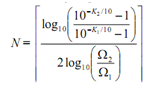
 Example: What is the order and transfer function of the analog Butterworth filter that satisfies the following specification?

Ω₁ = 200 rad/sec  K₁ = - 1 dB

    Ω₂= 600 rad/sec  K₂ = - 30 dB

Solution The order N is given by
 


Now, as in an earlier example, locate 8 poles uniformly on the unit circle, making sure to satisfy all the requirements ... and write down the transfer function, H(s), of the normalized

Butterworth filter (with a cut-off frequency of 1 rad/sec),
 
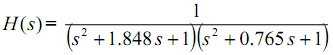
Next, determine the cutoff frequency Ω_c that corresponds to the given specifications and the order N = 4 determined above
 
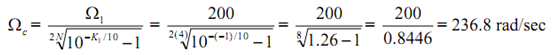
 Finally, we make the substitution  in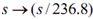 

H(s) and thereby move the cutoff frequency from 1 rad/sec to 236.8 rad/sec resulting in the transfer function H_a(s)

 

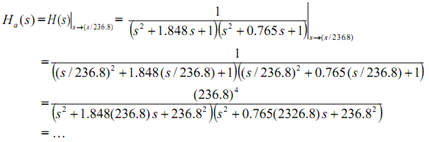
The more general Nth order Butterworth filter has the magnitude response given by
 

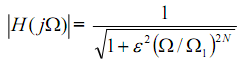
The parameter ε has to do with pass band attenuation and Ω₁ is the pass band edge frequency (not necessarily the same as the 3 dB cut-off frequency Ω_c).  

Email based Determining the order and transfer function assignment help - Determining the order and transfer function homework help at Expertsmind

Are you finding answers for Determining the order and transfer function based questions? Ask Determining the order and transfer function questions and get answers from qualified and experienced  Digital signal processing tutors anytime from anywhere 24x7. We at www.expertsmind.com offer Determining the order and transfer function assignment help -Determining the order and transfer function homework help and  Digital signal processing  problem's solution with step by step procedure.

Why Expertsmind for Digital signal processing assignment help service

1.     higher degree holder and experienced tutors

2.     Punctuality and responsibility of work

3.     Quality solution with 100% plagiarism free answers

4.     On Time Delivery

5.     Privacy of information and details

6.     Excellence in solving Digital signal processing queries in excels and word format.

7.     Best tutoring assistance 24x7 hours