Example 

The gain of a amplifier as a function of frequency is A (jω) = -16 x 106 / jω. A feedback path connected around it has β(j ω ) = 103 / (20 x 103 + jω )2. Will the oscillation take place in the system? If so, at then at what frequency ?

Solution:

The loop gain is  

To determine, if system will oscillate, we will first determine its frequency, if any, at which the phase angle of 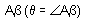  equals to 0° or a multiple of 360°. By using phasor algebra, we have

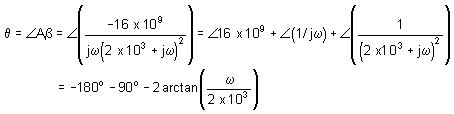
This expression will equal -360° if                                       ,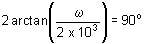

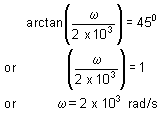
Therefore, the phase shift around the loop is -360° at ω = 2000 rad/s. We should now check to see if the gain magnitude |A β| = 1 at ω = 2 x 10³. The gain magnitude is given by

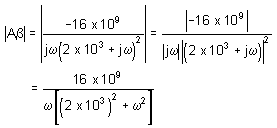
Substituting ω = 2 x 10³, we get

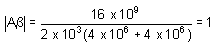
Thus, the Barkhausen criterion is satisfied at ω = 2 x 10³  rad/s and oscillation occurs at that frequency (2 x 10³ / 2 π= 318 .3 Hz).

Email based Electronics Devices and circuits assignment help - homework help at Expertsmind

Are you searching Electronics Engineering assignment help expert for help with Calculation of oscillations questions?  Calculation of oscillations topic is not easier to learn without any external help?  We at www.expertsmind.com offers free lecture notes for Electronics Devices and circuits assignment help and Electronics Devices and circuits homework help. Live tutors are available 24x7 hours for helping students in their Calculation of oscillations related problems. We provide step by step Calculation of oscillations question's answers with 100% plagiarism free content. We prepare quality content and notes for Calculation of oscillations topic under Electronics Devices and circuits theory and study material. These are avail for subscribed users and they can get advantages anytime.

Why Expertsmind for assignment help

1. Higher degree holder and experienced experts network
2. Punctuality and responsibility of work
3. Quality solution with 100% plagiarism free answers
4. Time on Delivery
5. Privacy of information and details
6. Excellence in solving electronics engineering questions in excels and word format.
7. Best tutoring assistance 24x7 hours